Professional Courses
Industry-relevant training in Business, Technology, and Design to help professionals and graduates upskill for real-world careers.
Categories
Interactive Games
Fun, engaging games to boost memory, math fluency, typing speed, and English skillsβperfect for learners of all ages.
Typing
Memory
Math
English Adventures
Knowledge
Enroll to start learning
Youβve not yet enrolled in this course. Please enroll for free to listen to audio lessons, classroom podcasts and take mock test.
Interactive Audio Lesson
Listen to a student-teacher conversation explaining the topic in a relatable way.
Understanding Algebraic Identities
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson
Welcome everyone! Today we're discussing algebraic identities. Can anyone tell me what an algebraic identity is?
Is it a type of equation that is always true?
Exactly! An algebraic identity is an equation that holds for all values of the variables involved. For example, \((x + y)^2 = x^2 + 2xy + y^2\) is an identity. Let's memorize this using the acronym PE for 'Perfect squares Equal'. Can anyone share any identities they remember?
Thereβs also \(x^2 - y^2 = (x + y)(x - y)\)!
Good job! That's Identity III. Remember, recognizing these identities helps us simplify expressions.
Application of Identities
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson
Now let's apply these identities. How would you find the product of \((x + 3)(x + 3)\)?
We can use Identity I, so it equals \((x + 3)^2\).
Correct! And whatβs the expanded form of that?
It would be \(x^2 + 6x + 9\).
Exactly! Let's remember it with the phrase 'Expand, Understand' each time we expand.
Factorization Using Identities
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson
Letβs now see how we can factor expressions using these identities. For instance, how would we factor \(49a^2 + 70ab + 25b^2\)?
I think we can compare it to Identity I!
Right! By recognizing the terms, we see it becomes \((7a + 5b)^2\).
So, it helps simplify the factorization process?
Yes, and a way to remember this is: 'Identify to Factor'.
More Complex Identities
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson
We can also extend these identities to three variables. Who can give me the squared form for three terms?
Thatβs \((x + y + z)^2 = x^2 + y^2 + z^2 + 2xy + 2yz + 2zx\)!
Awesome! We call this Identity V. For cubes, remember \((x + y)^3 = x^3 + y^3 + 3xy(x + y)\).
Can we make a memory aid for that?
Good thinking! Let's remember it with 'Third Time's Charm' for cubes.
Introduction & Overview
Read a summary of the section's main ideas. Choose from Basic, Medium, or Detailed.
Quick Overview
Standard
This section introduces several important algebraic identities, including the squares of binomials and cubic formulas. It also illustrates their applications in expanding, factoring, and calculating products using the identities.
Detailed
Algebraic Identities
Algebraic identities are equations that remain true regardless of the values of their variables. This section reviews key identities that include:
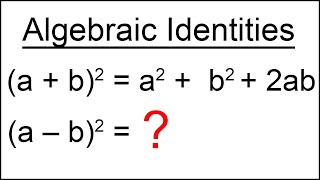
- Identity I: \((x + y)^2 = x^2 + 2xy + y^2\)
- Identity II: \((x - y)^2 = x^2 - 2xy + y^2\)
- Identity III: \(x^2 - y^2 = (x + y)(x - y)\)
- Identity IV: \((x + a)(x + b) = x^2 + (a + b)x + ab\)
The section includes examples demonstrating how to find products using these identities and emphasizes their utility for both expansion and factorization. Additionally, more complex identities such as those involving three variables and cubes of binomials are introduced, expanding the toolbox of algebraic techniques.
Example
Factorise:
Let
\( p(x) = x^3 - 25x^2 + 156x - 150. \)
We will also look for all the factors of \(-150\). Some of these are \( \pm 1, \pm 2, \pm 3, \pm 5, \pm 6, \pm 10, \pm 15, \pm 25, \pm 30, \pm 50, \pm 75, \pm 150\).
By trial, we find that \( p(1) = 0 \). So, \( 1 \) is a factor of \( p(x) \).
Now we see that \( x^3 - 25x^2 + 156x - 150 = (x - 1)(x^2 - 24x + 150) \) \[ (Why?) \]
We could have also got this by dividing \( p(x) \) by \( (x - 1) \) using the Factor theorem. By splitting the middle term, we have:
$$ \ x^2 - 24x + 150 = (x - 10)(x - 15) $$
So,
\[ p(x) = (x - 1)(x - 10)(x - 15) \]
Similar Question:
Factorise:
Let
\( p(x) = x^3 - 30x^2 + 195x - 270. \)
We will also look for all the factors of \(-270\). Some of these are \( \pm 1, \pm 2, \pm 3, \pm 5, \pm 6, \pm 9, \pm 10, \pm 15, \pm 18, \pm 27, \pm 30, \pm 45, \pm 90, \pm 135, \pm 270 \).
By trial, we find that \( p(3) = 0 \). So, \( 3 \) is a factor of \( p(x) \).
Now we see that \( x^3 - 30x^2 + 195x - 270 = (x - 3)(x^2 - 27x + 90) \) \[ (Why?) \]
We could have also got this by dividing \( p(x) \) by \( (x - 3) \) using the Factor theorem. By splitting the middle term, we have:
$$ \ x^2 - 27x + 90 = (x - 9)(x - 10) $$
So,
\[ p(x) = (x - 3)(x - 9)(x - 10) \]
Note: Ensure to verify all factor pairs and confirm through substitution to maintain solution integrity.
Youtube Videos
Audio Book
Dive deep into the subject with an immersive audiobook experience.
Definition of Algebraic Identities
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
From your earlier classes, you may recall that an algebraic identity is an algebraic equation that is true for all values of the variables occurring in it.
Detailed Explanation
An algebraic identity is a statement that equates two algebraic expressions and holds true for every possible value of the variables in these expressions. This means that if you substitute any value into the identity, both sides will give the same result. For example, the identity (x + y)Β² = xΒ² + 2xy + yΒ² works for any numbers you choose for x and y.
Examples & Analogies
Think of an algebraic identity like a recipe that works every time. Just like you can substitute different ingredients and still produce a delicious cake, you can change the values of the variables in an identity and it will still hold true, just like the recipe's instructions produce the same result.
Common Algebraic Identities
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
You have studied the following algebraic identities in earlier classes:
Identity I : (x + y)Β² = xΒ² + 2xy + yΒ²
Identity II : (x β y)Β² = xΒ² β 2xy + yΒ²
Identity III : xΒ² β yΒ² = (x + y)(x β y)
Identity IV : (x + a)(x + b) = xΒ² + (a + b)x + ab.
Detailed Explanation
Here are some common algebraic identities:
1. Identity I states the expansion of the square of a sum.
2. Identity II is the expansion of the square of a difference.
3. Identity III shows the difference of squares and how it can be factored into two binomials.
4. Identity IV allows us to expand the product of two binomials.
These identities are foundational tools in algebra that help simplify expressions and solve equations.
Examples & Analogies
Consider these identities like shortcuts in a manual. Instead of going through all the steps to get to a final product (the solution), these identities help you quickly get there without getting lost in unnecessary calculations. They are like knowing a direct route in a city that saves time.
Applying Identities in Examples
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Example 1: Find the following products using appropriate identities:
(i) (x + 3)(x + 3)
(ii) (x β 3)(x + 5)
Solution: (i) Here we can use Identity I: (x + y)Β² = xΒ² + 2xy + yΒ². Putting y = 3 in it,
(x + 3)(x + 3) = (x + 3)Β² = xΒ² + 2(x)(3) + (3)Β² = xΒ² + 6x + 9
(ii) Using Identity IV, we have (x β 3)(x + 5) = xΒ² + (β3 + 5)x + (β3)(5) = xΒ² + 2x β 15.
Detailed Explanation
In these examples, we see how to apply the identities to simplify products:
1. For (x + 3)(x + 3), we recognize it as (x + 3)Β² and use Identity I to expand it.
2. For (x β 3)(x + 5), we utilize Identity IV to expand by substituting the appropriate values. This saves a lot of time and effort versus multiplying out each term.
Examples & Analogies
Imagine you are packing boxes. Instead of figuring out how many boxes you need for every single item, you use the identities like a packing formula that quickly tells you how many full boxes can be packed together. This not only makes the task faster but also ensures accuracy in your packing.
Factoring with Identities
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
You have seen some uses of the identities listed above in finding the product of some given expressions. These identities are useful in factorisation of algebraic expressions also, as you can see in the following examples.
Example 13: Factorise:
(i) 49aΒ² + 70ab + 25bΒ²
(ii) 4yΒ² β 4y + 1.
Detailed Explanation
In these examples, we apply our identities to factor algebraic expressions:
1. For 49aΒ² + 70ab + 25bΒ², we recognize it as the expanded form of Identity I. We find that it factors to (7a + 5b)Β².
2. For 4yΒ² β 4y + 1, we can see this fits another identity and factors to (2y β 1)Β². These steps show how identities can help re-write expressions in a simpler form.
Examples & Analogies
Consider factoring algebraic expressions like peeling an onion to get to the core. Just as you carefully peel off layers to reach the center, using identities allows you to simplify complex expressions from their outer layer back to their basic components.
Extending Identities to Trinomials
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
So far, all our identities involved products of binomials. Let us now extend the Identity I to a trinomial x + y + z.
Detailed Explanation
We can extend our understanding of identities by exploring trinomials. For example, when computing (x + y + z)Β², we use the idea that we can first combine x and y, then add z to explore the expansion systematically. This allows us to create a new identity: Identity V: (x + y + z)Β² = xΒ² + yΒ² + zΒ² + 2xy + 2yz + 2zx.
Examples & Analogies
Think of this process as organizing a group project. At first, you might group team members to tackle individual tasks. Once each pair works, you combine results to get the final output, similar to how we combine different terms to reach a more comprehensive identity when dealing with three variables.
Further Examples of Identities
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Example 14: Write (3a + 4b + 5c)Β² in expanded form.
Solution:
Using Identity V, we have (3a + 4b + 5c)Β² = (3a)Β² + (4b)Β² + (5c)Β² + 2(3a)(4b) + 2(4b)(5c) + 2(5c)(3a).
Example 15: Expand (4a β 2b β 3c)Β².
Detailed Explanation
In these examples, we see how to apply the trinomial identity to obtain expanded forms. For (3a + 4b + 5c)Β², we follow Identity V to expand it to 9aΒ² + 16bΒ² + 25cΒ² + 24ab + 40bc + 30ac. Similarly, (4a β 2b β 3c)Β² also applies Identity V correctly by substituting negative values.
Examples & Analogies
Imagine planning a big event. You can think of the expanded form like organizing food, venues, decorations, and music into sections to ensure that every detail is covered, succinctly bringing together every part of the event into a coherent plan, just like the terms in the expanded form.
Exploring Cubic Identities
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
So far, we have dealt with identities involving second degree terms. Now let us extend Identity I to compute (x + y)Β³.
Detailed Explanation
We start by using the square expansion and then multiplying it by (x + y) to extend into cubic terms. This leads us to Identity VI: (x + y)Β³ = xΒ³ + yΒ³ + 3xy(x + y). We can also see how applying the negative of y gives us Identity VII: (x β y)Β³.
Examples & Analogies
Think of cubic identities like building a three-dimensional space. Just as every room in a house has dimensions that relate to each other, cubic identities relate the variables in a point-by-point expansion, demonstrating how different factors interact in three dimensions.
Application of Cubic Identities
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Example 17: Write the following cubes in the expanded form:
(i) (3a + 4b)Β³
(ii) (5p β 3q)Β³.
Detailed Explanation
In these examples, we leverage cubic identities for efficient calculations. For (3a + 4b)Β³, we identify x = 3a and y = 4b, using Identity VI to expand it to 27aΒ³ + 64bΒ³ + 108aΒ²b + 144abΒ². For (5p β 3q)Β³, we apply Identity VII for a similar expansion approach.
Examples & Analogies
Imagine packing three-dimensional boxes with various items. Writing out the expanded form is like noting down the capacity and arrangement of these boxes, ensuring all possible combinations are counted, similar to how we expand variables in algebraic forms.
Evaluating and Factorizing Using Identities
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Example 19: Factorise 8xΒ³ + 27yΒ³ + 36xΒ²y + 54xyΒ².
Solution: (2x)Β³ + (3y)Β³ + 3(2x)Β²(3y) + 3(2x)(3y)Β² = (2x + 3y)Β³.
Detailed Explanation
Here, we recognize the structure of the cubic identity in evaluating the factorization. By rearranging and applying the identities accordingly, we simplify a complex polynomial into a neat cubic factor.
Examples & Analogies
Think of evaluating and factorizing using identities as organizing your closet. When everything is neatly categorized, finding an outfit becomes simple. Similarly, using identities neatly organizes the polynomial, making it easier to factor and reveal its foundational structure.
Concluding Remark on Identities
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
You have learned various identities that can help you not only solve equations but also understand how polynomials interact through their structures.
Detailed Explanation
The study of algebraic identities unlocks deeper comprehension in mathematics, helping us manipulate polynomial expressions effectively. By recognizing patterns and applying these identities, we can simplify and solve problems more efficiently.
Examples & Analogies
Understanding these identities is like learning a new language. Once you know the keywords and grammar (the respective identities), you can communicate more effectively and understand conversations (equations and expressions) with greater clarity.
Definitions & Key Concepts
Learn essential terms and foundational ideas that form the basis of the topic.
Key Concepts
-
Algebraic Identities: Fundamental algebraic equations that hold true for any value.
-
Factorization: The process of writing an expression as a product of its factors.
-
Expansion: Rewriting an expression in an expanded or simplified manner.
Examples & Real-Life Applications
See how the concepts apply in real-world scenarios to understand their practical implications.
Examples
-
Using Identity I: \((x + 3)(x + 3) = (x + 3)^2 = x^2 + 6x + 9\)
-
Factoring: \(49a^2 + 70ab + 25b^2 = (7a + 5b)^2\)
Memory Aids
Use mnemonics, acronyms, or visual cues to help remember key information more easily.
π΅ Rhymes Time
-
When x and y do combine, it's squared, two xy is divine!
π Fascinating Stories
-
Imagine two friends x and y; when they hug, they become their own square.
π§ Other Memory Gems
-
FORESIGHT: Factor, Organize, Rearrange, Expand, Simplify, Highlight Terms.
π― Super Acronyms
SCOOPS
- Squares
- Cubes
- Operations
- Products
- Simplifications.
Flash Cards
Review key concepts with flashcards.
Glossary of Terms
Review the Definitions for terms.
-
Term: Algebraic Identity
Definition:
An algebraic equation that remains true for all values of its variables.
-
Term: Expansion
Definition:
The process of rewriting an expression in an extended form.
-
Term: Factorization
Definition:
The process of breaking down an expression into its components.