8 - QUADRILATERALS

Interactive Audio Lesson

Listen to a student-teacher conversation explaining the topic in a relatable way.

Introduction to Parallelograms

Unlock Audio Lesson

Signup and Enroll to the course for listening the Audio Lesson

Teacher
Teacher

Welcome, class! Today we're diving into quadrilaterals, specifically parallelograms. Who can tell me how many sides does a quadrilateral have?

Student 1
Student 1

It has four sides!

Teacher
Teacher

That's correct! A parallelogram is a specific case of a quadrilateral where both pairs of opposite sides are parallel. Remember that with the acronym 'OPPOSITE': Opposite sides are parallel, and the Opposite angles are equal. Let's get started with a fun activity.

Student 3
Student 3

What activity are we doing?

Teacher
Teacher

Great question! We'll cut out parallelograms from paper and explore their properties. This leads us to our first theorem: 'A diagonal of a parallelogram divides it into two congruent triangles.'

Congruence of Triangles

Unlock Audio Lesson

Signup and Enroll to the course for listening the Audio Lesson

Teacher
Teacher

Continuing from our previous activity, we discover that after cutting the parallelogram along the diagonal, we see two triangles. What do you think is true about these triangles?

Student 2
Student 2

I think they are congruent because they match up perfectly!

Teacher
Teacher

Exactly! This leads us to Theorem 8.1, which states that a diagonal divides a parallelogram into two congruent triangles. It's confirmed using the Alternate Interior Angles Theorem. Can anyone remind me why?

Student 4
Student 4

Because alternate angles formed between the transversals are equal when the lines are parallel!

Teacher
Teacher

Well done! Let's summarize the key point here: whenever you see a diagonal in a parallelogram, you can conclude that the triangles formed are congruent.

Properties of Opposite Sides and Angles

Unlock Audio Lesson

Signup and Enroll to the course for listening the Audio Lesson

Teacher
Teacher

Now let’s measure the opposite sides of our parallelogram. What do you observe?

Student 1
Student 1

They are equal!

Teacher
Teacher

Right! This illustrates Theorem 8.2, which states that in a parallelogram, opposite sides are equal. There’s another theorem about opposite angles. What do we discover when we measure them?

Student 3
Student 3

They are equal too!

Teacher
Teacher

Correct! Remember, the acronym 'OA' stands for 'Opposite Angles', which are equal in parallelograms. Summarizing, we’ve established that both the opposite sides and opposite angles are equal.

Diagonals in Parallelograms

Unlock Audio Lesson

Signup and Enroll to the course for listening the Audio Lesson

Teacher
Teacher

Next, let's explore diagonals. What happens when we draw both diagonals in a parallelogram?

Student 2
Student 2

They intersect at the midpoint!

Teacher
Teacher

Exactly! This leads us to Theorem 8.6 about diagonals bisecting each other. To remember this concept, you could think of the mnemonic 'BIS' for 'Bisection In Set'. How does the knowledge of bisected diagonals help us?

Student 4
Student 4

It helps us prove that a quadrilateral is a parallelogram if its diagonals bisect each other!

Teacher
Teacher

Fantastic! That brings us to Theorem 8.7. We can now affirm any quadrilateral with bisected diagonals is indeed a parallelogram.

Special Cases: Rectangles and Rhombuses

Unlock Audio Lesson

Signup and Enroll to the course for listening the Audio Lesson

Teacher
Teacher

Lastly, let’s discuss rectangles and rhombuses. Can anyone explain the distinct characteristics of a rectangle?

Student 1
Student 1

A rectangle has four right angles!

Teacher
Teacher

Correct! A rectangle is also a special type of parallelogram. And what about a rhombus?

Student 3
Student 3

In a rhombus, the diagonals are perpendicular!

Teacher
Teacher

Right again! Theorems relating to rectangles and rhombuses build on our understanding of basic parallelograms. Summarize it all: what do parallelograms, rectangles, and rhombuses have in common?

Student 4
Student 4

They all have opposite sides that are parallel and equal!

Teacher
Teacher

Excellent! Remember to keep these core properties in mind, as they apply to many geometric shapes.

Introduction & Overview

Read a summary of the section's main ideas. Choose from Basic, Medium, or Detailed.

Quick Overview

The section delves into the properties of parallelograms, including congruence of triangles formed by diagonals, equal opposite sides and angles, and the unique characteristics of rectangles and rhombuses.

Standard

This section explores the definition and properties of parallelograms, including the congruence of triangles created by diagonals, equal opposite sides and angles, and the specific properties of rectangles and rhombuses. It emphasizes theorems relating to these properties, with engaging activities and examples to reinforce understanding.

Detailed

Detailed Summary of Quadrilaterals

In this section, we focus on parallelograms, which are defined as quadrilaterals with two pairs of parallel sides. The key points covered include:

Properties of Parallelograms

  • Congruent Triangles: A diagonal of a parallelogram divides it into two congruent triangles. This is proved using alternate angles and corresponding parts of congruent triangles.
  • Equal Opposite Sides: It is demonstrated that in parallelograms, opposite sides are equal, validated through the aforementioned triangle congruence.
  • Equal Opposite Angles: Measuring angles shows that opposite angles of a parallelogram are equal, and the converse is also proven.
  • Bisecting Diagonals: Both diagonals in a parallelogram bisect each other at their midpoint, and the converse is similarly proven.

Special Cases: Rectangles and Rhombuses

  • A rectangle is characterized as a parallelogram with one right angle, leading to the conclusion that all angles are right angles.
  • For rhombuses, the properties include that the diagonals are perpendicular to each other.

Theoretical Applications

  • The section also encourages engaging activities to observe these properties in practice, and includes exercises to reinforce students' understanding of the theorems.

In conclusion, understanding the properties of parallelograms not only lays a crucial foundation for further geometry concepts but also enhances students' spatial reasoning and problem-solving skills.

Youtube Videos

Quadrilaterals Class 9 in One Shot 🔥 | Class 9 Maths Chapter 8 Complete Lecture | Shobhit Nirwan
Quadrilaterals Class 9 in One Shot 🔥 | Class 9 Maths Chapter 8 Complete Lecture | Shobhit Nirwan
Quadrilaterals | Introduction | Chapter 8 | SEED 2024-2025
Quadrilaterals | Introduction | Chapter 8 | SEED 2024-2025
CBSE Class 9 || Maths || Quadrilateral || Animation || in English @digitalguruji3147
CBSE Class 9 || Maths || Quadrilateral || Animation || in English @digitalguruji3147
Quadrilateral Class 9 One Shot Revision in 15 Min | Class 9 Maths Chapter 8
Quadrilateral Class 9 One Shot Revision in 15 Min | Class 9 Maths Chapter 8
Quadrilateral class 9 problem
Quadrilateral class 9 problem
🔥Class-9th | Math's | CH- 7,8,9 | All most Expected Question Ever| #Cbse 9th #live
🔥Class-9th | Math's | CH- 7,8,9 | All most Expected Question Ever| #Cbse 9th #live
Theorem:8.5 Chapter:8 Quadrilaterals | Ncert Maths Class 9
Theorem:8.5 Chapter:8 Quadrilaterals | Ncert Maths Class 9
7 Types of Quadrilaterals
7 Types of Quadrilaterals
Chapter:8 (Introduction) Quadrilaterals | Ncert Maths Class 9 | Cbse.
Chapter:8 (Introduction) Quadrilaterals | Ncert Maths Class 9 | Cbse.
class 8 math chapter 3 Understanding quadrilateral. mcq questions..
class 8 math chapter 3 Understanding quadrilateral. mcq questions..

Audio Book

Dive deep into the subject with an immersive audiobook experience.

Properties of a Parallelogram

Unlock Audio Book

Signup and Enroll to the course for listening the Audio Book

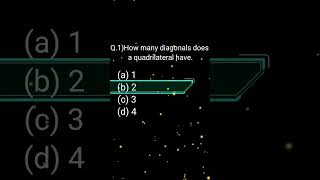
You have already studied quadrilaterals and their types in Class VIII. A quadrilateral has four sides, four angles and four vertices. A parallelogram is a quadrilateral in which both pairs of opposite sides are parallel.

Detailed Explanation

A parallelogram is a special type of quadrilateral with two important properties: each pair of opposite sides is parallel. This means that the sides that face each other do not intersect and stay the same distance apart. Because they are parallel, when you move one side, the other will also shift in the same direction, keeping them aligned.

Additionally, a quadrilateral is defined as a shape that has four sides and four angles, which applies to all parallelograms as well.

Examples & Analogies

Imagine two train tracks running parallel to each other. As the train moves along one track, the other track continues to run alongside it without ever meeting. Similarly, the opposite sides of a parallelogram behave like these parallel tracks.

Activity: Exploring Congruent Triangles

Unlock Audio Book

Signup and Enroll to the course for listening the Audio Book

Cut out a parallelogram from a sheet of paper and cut it along a diagonal. You obtain two triangles. What can you say about these triangles? Place one triangle over the other. Turn one around, if necessary. What do you observe? Observe that the two triangles are congruent to each other.

Detailed Explanation

In this activity, when you cut a parallelogram along one of its diagonals, you create two triangles. If you place one triangle over the other, you will notice they match perfectly, demonstrating that they are congruent. Congruent triangles have the same size and shape, meaning all their corresponding sides and angles are equal.

Examples & Analogies

Think of a cake that you cut in half. If you pick up one half and place it directly over the other half, they fit exactly. This is similar to how the two triangles fit perfectly when you observe they are congruent.

Theorem 8.1: Diagonal of a Parallelogram

Unlock Audio Book

Signup and Enroll to the course for listening the Audio Book

Theorem 8.1: A diagonal of a parallelogram divides it into two congruent triangles. Proof: Let ABCD be a parallelogram and AC be a diagonal. The diagonal AC divides parallelogram ABCD into two triangles, namely, ∆ ABC and ∆ CDA.

Detailed Explanation

This theorem states that when you draw a diagonal in a parallelogram, it splits it into two triangles that are congruent, meaning they are identical in shape and size. The proof involves showing that certain angles are equal (by using properties of parallel lines) and that a side in each triangle is the same, allowing us to conclude that the triangles are congruent based on the ASA (Angle-Side-Angle) rule.

Examples & Analogies

If you take a rectangular piece of paper (which is a type of parallelogram) and fold it diagonally, the two halves will overlap perfectly. This overlapping represents the congruent triangles formed by the diagonal.

Theorem 8.2: Opposite Sides of a Parallelogram

Unlock Audio Book

Signup and Enroll to the course for listening the Audio Book

Theorem 8.2: In a parallelogram, opposite sides are equal.

Detailed Explanation

This theorem emphasizes another key property of parallelograms: opposite sides are equal in length. This is critical because it reinforces the idea that parallelograms are symmetrical; every action you take on one side influences the opposite side equally.

Examples & Analogies

Think about a balanced seesaw. When one side goes up, the other must go down to keep it balanced, just like the opposite sides of a parallelogram being equal.

Theorem 8.3: Converse of Theorem 8.2

Unlock Audio Book

Signup and Enroll to the course for listening the Audio Book

Theorem 8.3: If each pair of opposite sides of a quadrilateral is equal, then it is a parallelogram.

Detailed Explanation

This theorem proposes a converse condition: if you have a quadrilateral and you know that each pair of its opposite sides are equal, then you can conclude it is a parallelogram. The importance of understanding both direct and converse statements helps solidify your knowledge of how geometrical properties interrelate.

Examples & Analogies

Imagine a rectangle where you measure all sides. If you find that the opposite sides are equal, you can confidently say you're dealing with a parallelogram, much like identifying a family resemblance in traits.

Theorems on Angles in a Parallelogram

Unlock Audio Book

Signup and Enroll to the course for listening the Audio Book

Theorem 8.4: In a parallelogram, opposite angles are equal. Theorem 8.5: If in a quadrilateral, each pair of opposite angles is equal, then it is a parallelogram.

Detailed Explanation

These theorems indicate that, just as the opposite sides of a parallelogram are equal, so are the opposite angles. The first theorem confirms this property, while the second theorem provides a converse: if you find a quadrilateral in which the opposite angles are equal, it must necessarily be a parallelogram.

Examples & Analogies

Visualize a pair of scissors: when one blade is pushed down, the other rises towards the same angle. This shows how equal angles work in harmony, similar to opposite angles in a parallelogram.

Diagonals of a Parallelogram

Unlock Audio Book

Signup and Enroll to the course for listening the Audio Book

Theorem 8.6: The diagonals of a parallelogram bisect each other. Theorem 8.7: If the diagonals of a quadrilateral bisect each other, then it is a parallelogram.

Detailed Explanation

This section explains that the diagonals of a parallelogram not only divide the shape into two triangles, but they also cut each other in half. The converse theorem affirms that if you have a quadrilateral where the diagonals bisect each other, it guarantees that the shape is a parallelogram.

Examples & Analogies

Consider a kite where the strings meet at the center. Each string bisects the other at that point, reflecting how the diagonals in a parallelogram behave.

Examples Involving Parallelograms

Unlock Audio Book

Signup and Enroll to the course for listening the Audio Book

Examples demonstrating properties of parallelograms including rectangles and rhombuses.

Detailed Explanation

In this section, specific examples like rectangles and rhombuses are used to illustrate how the properties of parallelograms manifest in recognizable shapes. Each example showcases a unique property, such as angles and sides, helping to reinforce understanding.

Examples & Analogies

Think about how real-life objects like windows and tables exhibit the properties of parallelograms. By observing these standard shapes, you can better visualize the theoretical concepts discussed here.

Definitions & Key Concepts

Learn essential terms and foundational ideas that form the basis of the topic.

Key Concepts

  • Congruent Triangles: A diagonal of a parallelogram creates two congruent triangles.

  • Opposite Sides: Opposite sides of a parallelogram are equal.

  • Opposite Angles: Opposite angles of a parallelogram are equal.

  • Bisecting Diagonals: The diagonals of a parallelogram bisect each other.

  • Special Properties: Rectangles have right angles; rhombuses have equal sides.

Examples & Real-Life Applications

See how the concepts apply in real-world scenarios to understand their practical implications.

Examples

  • Cutting a parallelogram along its diagonal demonstrates that the resulting triangles are congruent.

  • Measuring the opposite sides of a parallelogram shows they are equal, confirming Theorem 8.2.

  • Observing that the diagonals of a parallelogram bisect each other provides a visual understanding of Theorem 8.6.

Memory Aids

Use mnemonics, acronyms, or visual cues to help remember key information more easily.

🎵 Rhymes Time

  • In a parallelogram, not a scam, opposite sides are always the same!

📖 Fascinating Stories

  • Once upon a time in a land of shapes, a parallelogram discovered its secrets; opposite sides and angles lived harmoniously equal. Will they find their area together?

🧠 Other Memory Gems

  • Remember 'B.O.B.' for Bisecting Opposite Bisectors - capture the essence of diagonals bisecting and discovering the properties they hold.

🎯 Super Acronyms

OPEA

  • Opposite Sides Equal
  • Angles Equal – the key traits of parallelograms!

Flash Cards

Review key concepts with flashcards.

Glossary of Terms

Review the Definitions for terms.

  • Term: Parallelogram

    Definition:

    A quadrilateral with both pairs of opposite sides parallel.

  • Term: Congruent

    Definition:

    Two figures that have the same shape and dimensions.

  • Term: Diagonals

    Definition:

    Line segments connecting opposite vertices of a polygon.

  • Term: Alternate Angles

    Definition:

    Angles formed on opposite sides of a transversal intersecting two lines.

  • Term: Rectangle

    Definition:

    A parallelogram with four right angles.

  • Term: Rhombus

    Definition:

    A parallelogram with all four sides of equal length.

  • Term: ASA Rule

    Definition:

    Angle-Side-Angle rule for proving triangle congruency.