Professional Courses
Industry-relevant training in Business, Technology, and Design to help professionals and graduates upskill for real-world careers.

Categories
Interactive Games
Fun, engaging games to boost memory, math fluency, typing speed, and English skills—perfect for learners of all ages.

Typing
Memory
Math
English Adventures
Knowledge
Enroll to start learning
You’ve not yet enrolled in this course. Please enroll for free to listen to audio lessons, classroom podcasts and take mock test.
Interactive Audio Lesson
Listen to a student-teacher conversation explaining the topic in a relatable way.
Understanding the Median
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson

Today, we are going to discuss the median, a crucial measure of central tendency. The median is essentially the middle value of a dataset when it is organized in ascending order.

How is that different from the mean?

Good question! Unlike the median, the mean takes all values into account and can be affected by extreme values. The median, however, focuses solely on the middle point.

So, when do we use the median instead of the mean?

We typically use the median when dealing with skewed distributions or when outliers are present, as it gives a better representation of a typical value.

Remember the acronym 'MIDDLE' - Median Is The Limit of Data Less Extreme!

That's a great way to remember it!

Let's summarize. The median represents the central point of data and is less sensitive to outliers. Next, we’ll see how to calculate the median from grouped data.
Cumulative Frequency
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson

To find the median in grouped data, we first need to create a cumulative frequency table.

What exactly is a cumulative frequency?

Cumulative frequency represents the total number of observations that fall below the upper limit of each class interval.

Can you show us how to compute it?

Sure! Let's take an example where we have scores. We can keep a running total as we go down the table of frequency counts.

So, the last entry in the cumulative frequency table represents the total number of observations?

Exactly! Now remember the mnemonic 'CUMULATIVE' - Count Until Much Ultimately Leads to Average Total in Incremental Values.

Now, let’s work on understanding where the median comes from using cumulative frequencies.
Finding the Median Class
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson

To find the median, we first identify the median class from the cumulative frequency table.

How do we find that?

We calculate n divided by 2. If our total n is 53, then n/2 is 26.5. We look for the cumulative frequency just greater than this value.

I see, so we check the cumulative frequencies until we surpass 26.5.

Correct! Remember the phrase 'Above and Beyond'—we look for the next cumulative frequency that surpasses half.

Is this where we decide which class contains the median?

Exactly! Once we find that class, we can apply the median formula.
Median Formula
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson

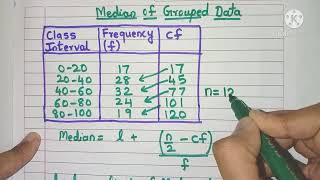
Now, let’s explore the median formula. The median is calculated with the formula: Median = l + ((n/2 - cf) / f) * h.

Can you break that down for us?

Certainly! Here, l is the lower limit of the median class, cf is the cumulative frequency of the class preceding it, f is the frequency of the median class, and h is the width of the class interval.

What if the class sizes are not the same?

Good observation! As discussed, the formula adjusts accordingly, but we will stick to equal sizes for now.

'FORMULA' can remind you: Find Our Right Measure Using Limits and Averages!

Thanks for that, it’s a clever way to remember!

Summarizing, we explored the median formula details and its components. Next, let's work through examples using this method.
Practical Examples
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson

Finally, we’ll put everything into practice by calculating the median using examples.

Can you show us one? I feel this will clear things up!

Absolutely! We will find the median for a set of student scores within specified groups.

I want to note the steps clearly!

"1. Create the frequency table.
Introduction & Overview
Read a summary of the section's main ideas. Choose from Basic, Medium, or Detailed.
Quick Overview
Standard
The section elaborates on the definition and significance of the median as a measure of central tendency for grouped data. It provides steps for constructing cumulative frequency distributions and calculating the median, including illustrative examples that clarify the process and formula used.
Detailed
Detailed Summary
This section details the concept of the median as a measure of central tendency, specifically for grouped data. The median represents the middle value when data points are organized in ascending order. In grouped data, which is presented in class intervals, direct observation of middle values is not feasible. Thus, the calculation involves constructing cumulative frequency tables.
- Finding the Median:
- Depending on whether the total number of observations (n) is odd or even, the position of the median can be established. If n is even, the median will be the average of the two middle values.
- For grouped data, cumulative frequency distributions are essential. The median class is determined by locating the cumulative frequency that is just greater than or equal to n/2.
-
Formula:
The median can be calculated using the formula:
\[
ext{Median} = l + \left(\frac{n/2 - cf}{f} \right) \times h
\]
Where:
- l = lower limit of the median class
- n = total number of observations
- cf = cumulative frequency of the class preceding the median class
- f = frequency of the median class
- h = width of class intervals.
Illustrations in the section demonstrate this procedure using cumulative frequency data to find the median for test scores and heights, underscoring the importance of this statistic in understanding data distributions in various contexts.
Youtube Videos








Audio Book
Dive deep into the subject with an immersive audiobook experience.
Introduction to Median
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
As you have studied in Class IX, the median is a measure of central tendency which gives the value of the middle-most observation in the data. Recall that for finding the median of ungrouped data, we first arrange the data values of the observations in ascending order. Then, if n is odd, the median is the n/2th observation. And, if n is even, then the median will be the average of the n/2th and the (n/2 + 1)th observations.
Detailed Explanation
The median is the value that separates a dataset into two equal halves. When you arrange the numbers in order, the median is the middle number. For an odd number of observations, it’s simply the middle number. For an even number of observations, it’s the average of the two middle numbers. This ensures that 50% of the values fall below the median and 50% fall above it, making it a useful measure of central tendency, especially when the data has outliers.
Examples & Analogies
Imagine a line of students waiting to board a bus. If there are 5 students, the one in the middle is the median student. If there are 6 students, we look at the two in the middle and average their heights to find the typical height of the group. Similar logic applies when setting up your grades in a school, where you want to find out if the majority scored above or below a certain mark.
Finding the Median Using a Frequency Table
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Suppose, we have to find the median of the following data, which gives the marks, out of 50, obtained by 100 students in a test:
Marks obtained: 20, 29, 28, 33, 42, 38, 43, 25
Number of students (Frequency): 6, 28, 24, 15, 2, 4, 1, 20.
Detailed Explanation
Firstly, a frequency table is constructed to organize the data. In this case, the marks are arranged in ascending order along with their respective frequencies. From the frequency table, we sum the frequencies to understand how many students fall within specific marks, facilitating the identification of the middle observation. When we total the frequencies, we find n = 100. Since this is even, we average the 50th and 51st observations in our ordered set to find the median value.
Examples & Analogies
Think of a classroom where students are lined up in order of height. If you want to find the height that divides the class evenly, you would start counting from both ends until you reach the middle person or persons. In a frequency table, it’s like finding out how many students fall below and above certain height thresholds, similar to finding the median height of students in a class.
Cumulative Frequency and Its Importance
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
To find the precise median, we create a cumulative frequency table that summarizes how many observations fall below certain marks, helping pinpoint the median class.
Detailed Explanation
Cumulative frequency accumulates the frequency counts giving a running total, which is crucial for determining the median. When we create a cumulative frequency table, we can easily identify the mark where half the students fall below or above, allowing us to find the median efficiently. This table helps visualize how many observations lie below certain cutoffs.
Examples & Analogies
Consider a bakery that tracks how many pastries are sold each hour. By keeping a cumulative tally, they can see how sales add up throughout the day. This is similar to cumulative frequency, where you keep a running total to figure out how many students scored below a certain mark.
Applying the Median Formula for Grouped Data
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
To find the median of grouped data, we can make use of cumulative frequency distributions. We will find the median class from the cumulative frequency table and apply the formula:
Median = l + [(n/2 - cf) / f] × h, where l = lower limit of median class, cf = cumulative frequency of class before median class, f = frequency of median class, and h = class width.
Detailed Explanation
The median formula for grouped data allows us to calculate the median when data is not in individual values but rather in group categories. We first identify the median class, which is where the cumulative frequency just exceeds n/2. Using this class, we substitute into the formula to find the median value, thereby pinpointing the exact position within the data grouping.
Examples & Analogies
Picture hosting a party where you want to find the average age of the attendees. Instead of asking everyone for their age individually, you group their ages into ranges (e.g., under 20, 20-30, etc.). The median tells you that half the people at your party are younger or older than a certain age, helping you plan activities suitable for your guests.
Example of Median Calculation
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Consider a grouped frequency distribution of marks obtained, out of 100, by 53 students:
Marks: 0 - 10, 10 - 20, 20 - 30, 30 - 40, 40 - 50, 50 - 60, 60 - 70, 70 - 80, 80 - 90, 90 - 100.
By identifying the median class and applying the formula, we can arrive at the median mark for these students.
Detailed Explanation
After setting up the frequency table and accumulating the frequencies, we find that n = 53. Therefore, n/2 = 26.5. The median class is determined to be the one with the cumulative frequency closest to and greater than 26.5. We use the median formula with this class to find the exact median mark, thus showing how many students scored below this mark.
Examples & Analogies
Think of it like a scoreboard in a game where you want to know the score that splits the players into two equal teams. The median lets you know the score where half of the team has scored below and half above, just like knowing the middle score among players helps understand overall performance.
Understanding the Importance of the Median
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
The median is particularly important in statistics as it provides a better measure of central tendency in data with outliers because it is not affected by extreme values unlike the mean.
Detailed Explanation
In datasets where there are extreme outliers, the median provides a more reliable measure of typical values. This is crucial in real-world problems where data can vary widely and skew the mean. By focusing on the median, we can better understand the actual central tendency of the more typical values in the data.
Examples & Analogies
Imagine someone examining income levels in a city. If a billionaire lives there, they might skew the average income very high, misleading the understanding of most people's earnings. However, the median income would provide a clearer picture of what the majority earn, highlighting the financial situation of the common person.
Definitions & Key Concepts
Learn essential terms and foundational ideas that form the basis of the topic.
Key Concepts
-
Median: The value dividing the higher half from the lower half of the data set.
-
Cumulative Frequency: The total of the frequencies from the start up to a certain class interval.
-
Median Class: The class interval where the median lies.
-
Class Interval: Specific ranges of grouped data.
Examples & Real-Life Applications
See how the concepts apply in real-world scenarios to understand their practical implications.
Examples
-
Example 1: Finding the median from a cumulative frequency table where class intervals represent annual incomes of households.
-
Example 2: Calculating the median height of school children from grouped height data.
Memory Aids
Use mnemonics, acronyms, or visual cues to help remember key information more easily.
🎵 Rhymes Time
-
To find the median, don't be a fool, Step by step, just follow the rule.
📖 Fascinating Stories
-
Imagine a race where runners line-up. The median is the runner in the middle who won’t be stuck!
🧠 Other Memory Gems
-
Median's Might: Middle values clear the sight!
🎯 Super Acronyms
M.A.C - Median As Center. Remember, that's how we find our key median value!
Flash Cards
Review key concepts with flashcards.
Glossary of Terms
Review the Definitions for terms.
-
Term: Median
Definition:
The middle value of a dataset when arranged in order; represents the 50th percentile.
-
Term: Cumulative Frequency
Definition:
The running total of frequencies up to a given class interval.
-
Term: Median Class
Definition:
The class interval that contains the median value in grouped data.
-
Term: Class Interval
Definition:
A range of values that grouped data can belong to.
-
Term: Frequency
Definition:
The number of occurrences of values in a class interval.

