1.2.6 - Distributivity of multiplication over addition for rational numbers
Enroll to start learning
You’ve not yet enrolled in this course. Please enroll for free to listen to audio lessons, classroom podcasts and take practice test.
Interactive Audio Lesson
Listen to a student-teacher conversation explaining the topic in a relatable way.
Understanding the Distributive Property
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

Today, we are going to explore an important property of multiplication called the Distributive Property. Who can tell me what they think it means?

Is it how multiplication works with addition, like when you can multiply a number by a sum?

Exactly! The distributive property states that a(b + c) = ab + ac. This means if you have a number a multiplied by the sum of b and c, you can distribute 'a' to both b and c. Let's use rational numbers to illustrate this.

Can we see a specific example?

Sure! Let’s use a = -3/4, b = 2/3, and c = -5/6. If we compute (-3/4) × (2/3 + -5/6), we can distribute it as follows. It will be: (-3/4) × (2/3) + (-3/4) × (-5/6).

So we multiply separately and then add the results?

Exactly! Great understanding!

Let’s recap today’s lesson: The distributive property helps us simplify multiplication over addition effectively.
Application of Distributive Property
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

Now, how do we use this property in solving problems? Can someone give an example problem?

What if I have to calculate -2 × (5 + 1)?

Great! Using the distributive property, we can rewrite it as -2 × 5 + -2 × 1. Can anyone calculate that?

That would be -10 + -2, so -12!

Correct! This shows how simplifying or expanding expressions can make the calculations easier and more efficient.

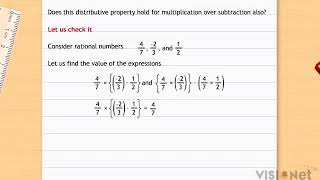
Does it work with subtraction too?

Absolutely! You can distribute over subtraction as well. For example, a(b - c) = ab - ac.

Let’s confirm what we learned today: Distributive property applies to both addition and subtraction.
Illustrating Zero and One
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

Let’s talk about how the numbers zero and one interact with the distributive property? What can we say about multiplying with zero?

If you multiply anything by zero, the answer is zero!

Correct! So, if we distributed a number multiplied by zero, say a(0 + b) would equal ab + 0? What does that simplify to?

That simplifies just to ab, right?

Exactly! Now, what happens when multiplying by one?

It stays the same! Like one is the identity for multiplication.

Yes, it respects the distributive property too! So remember both zero and one play special roles.

Today, we learned how zero and one relate to the distributive property.
Introduction & Overview
Read summaries of the section's main ideas at different levels of detail.
Quick Overview
Standard
In this section, the distributive property of multiplication over addition for rational numbers is discussed. Examples illustrate how multiplying a rational number by a sum can be expressed as the sum of products, and this property is crucial for simplifying expressions in algebra.
Detailed
Distributivity of Multiplication over Addition for Rational Numbers
The distributive property states that for all rational numbers a, b, and c, the following holds:
- Distributivity Over Addition: a(b + c) = ab + ac
- Distributivity Over Subtraction: a(b - c) = ab - ac
This means that if you have a rational number multiplied by the sum (or difference) of two other rational numbers, you can distribute the multiplication across the addition (or subtraction). This is a fundamental property in mathematics that enables the simplification and restructuring of expressions to make calculations easier and more manageable. For instance:
Given:
- a = -3/4, b = 2/3, c = -5/6
We can show the distributive property:
- Example:
- Calculate
(-3/4) × (2/3 + -5/6). - By distributive property:
(-3/4) × (2/3 + -5/6) = (-3/4) × (2/3) + (-3/4) × (-5/6). - Solve the two products separately and then add them together.
In this section, we explore various examples to make this property evident and reinforce its significance in mathematical operations involving rational numbers.
Youtube Videos









Audio Book
Dive deep into the subject with an immersive audiobook experience.
Introduction to Distributivity
Chapter 1 of 4
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
To understand this, consider the rational numbers −3/4, 2/3, and −5/6.
Detailed Explanation
Distributivity is a property that involves how multiplication interacts with addition. This property states that when you multiply a number by a sum, it is the same as multiplying each addend separately and then adding the results. In this case, we are looking at the rational numbers -3/4, 2/3, and -5/6 to demonstrate the property.
Examples & Analogies
Think of distributivity like sharing a pizza among friends. If you have three friends and you buy a pizza that costs $10, you can either calculate the total cost by multiplying $10 by 3 (for all the pizzas) or by splitting the pizza first into 3 equal slices, counting how much each person gets, and then figuring the total cost based on the slices they took.
Applying Distributivity
Chapter 2 of 4
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
−3 × (2/4 + −5/6) = −3 × (8/24 + −20/24) = −3 × (−12/24).
Detailed Explanation
In this step, we are applying the distributive property. We take −3 and multiply it by the sum of 2/4 and -5/6. The first step is to find a common denominator, which is 24 in this case, so we convert both fractions to have this denominator. After simplifying, we multiply −3 by the resulting fraction -12/24, which simplifies to -1/2.
Examples & Analogies
Imagine you're distributing snacks among two groups of friends. You have 12 cookies (representing the total value of -12 as a negative because it’s a loss in terms of costs). Distributing them evenly among your friends demonstrates how you can break down the process—each friend gets cookies separately just like we distributed values in math.
Final Calculation
Chapter 3 of 4
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
Therefore, −3 × (2/4 + −5/6) = −3 × 2/4 + −3 × −5/6 = 1/2.
Detailed Explanation
Finally, we compute separately the products after distributing the multiplication. We calculate the parts: -3 times 2/4 gives us a product of -3/2 and for -3 times -5/6 gives us positive results. Adding these results together gives us 1/2, concluding our property demonstration.
Examples & Analogies
Returning to our pizza analogy, if you started with a negative amount (like owing cookies) and then share them evenly, your friends could potentially trade cookies back and forth, balancing each other's snack supply to finally see what’s left, just as we balance equations in math.
General Formula of Distributivity
Chapter 4 of 4
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
For all rational numbers a, b, and c, a × (b + c) = ab + ac and a × (b - c) = ab - ac.
Detailed Explanation
The distributive property can be generalized. It means you can multiply any number by a sum or difference of other numbers and achieve the same result, allowing flexibility in computation. This formula helps simplify calculations and is foundational in algebra.
Examples & Analogies
Imagine you have a job that pays you weekly based on hours worked. If you work overtime, instead of calculating the full amount each time, you can just calculate it based on the basic hours and multiply that figure by both the usual work and the overtime, thus confirming you’re using distributivity to arrive at your final paycheck.
Key Concepts
-
Distributive Property: It allows multiplication to distribute over addition, making calculations easier.
-
Rational Numbers: Fractions that can be expressed as a ratio of two integers.
Examples & Applications
Example 1: a = -3/4, b = 2/3, c = -5/6. Calculate (-3/4) × (2/3 + -5/6)
Example 2: Using the distributive property, calculate -2 × (5 + 1) as -2 × 5 + -2 × 1.
Memory Aids
Interactive tools to help you remember key concepts
Rhymes
If you see a sum, and multiplication is near, distribute it well and have no fear!
Stories
Once there was a number, let's call it a, who had two friends b and c. A wanted to share its gifts equally with b and c, so it multiplied by their total; together, they became an even better sum.
Memory Tools
Remember: D for Distributive, A for Addition, S for Subtraction, helps us keep the numbers in the right function!
Acronyms
DAS
Distributive add then solve! An easy way to recall how to apply it.
Flash Cards
Glossary
- Distributive Property
A property that states a(b + c) = ab + ac, allowing multiplication to be distributed over addition or subtraction.
- Rational Number
A number that can be expressed as the quotient or fraction of two integers, where the denominator is not zero.
Reference links
Supplementary resources to enhance your learning experience.
