12.1 - Graphical Representation of Data

Interactive Audio Lesson

Listen to a student-teacher conversation explaining the topic in a relatable way.

Bar Graphs

Unlock Audio Lesson

Signup and Enroll to the course for listening the Audio Lesson

Teacher
Teacher

Today, we will learn about bar graphs. Can anyone tell me what a bar graph is?

Student 1
Student 1

Isn't it a graph that uses bars to show information?

Teacher
Teacher

Exactly! A bar graph uses rectangular bars to represent data. The height of each bar indicates the quantity of the respective category. For example, if we surveyed students about their favorite fruits, each type of fruit would have a bar representing the number of votes it received.

Student 2
Student 2

What do we place on the axes?

Teacher
Teacher

Good question, Student_2! The categories go on the x-axis, and the values are represented on the y-axis. For example, in the fruit survey, fruits would be on the x-axis, while the number of votes would be on the y-axis.

Student 3
Student 3

How do we decide how wide the bars should be?

Teacher
Teacher

The width of the bars can be uniform across the graph, and ensuring there's equal spacing helps maintain clarity. Remember, the phrase 'One picture is worth a thousand words' reflects the power of graphical representation!

Student 4
Student 4

Interesting! So, what should I remember about bar graphs?

Teacher
Teacher

Think 'B-A-R': Bars show Information and Relative values! Let’s summarize the key points. Who can recap what we learned today?

Histograms

Unlock Audio Lesson

Signup and Enroll to the course for listening the Audio Lesson

Teacher
Teacher

Now that we've covered bar graphs, let’s move to histograms. Does anyone know what makes histograms different?

Student 1
Student 1

Are they just like bar graphs but without spaces?

Teacher
Teacher

That's right! Histograms are used for continuous data and the bars touch each other, representing ranges rather than individual categories. For instance, you might create a histogram to represent the weight intervals of students.

Student 2
Student 2

How do we create the bars for a histogram?

Teacher
Teacher

To create a histogram, start by determining the range of your data. Each range or interval is used to create a bin. The height of each bar will correspond to how many data points fall within that range.

Student 3
Student 3

What if the intervals vary in size?

Teacher
Teacher

Great question, Student_3! In cases where the intervals vary, the area of each bar must be proportional to the frequency of data within the interval. This is key for accurately interpreting the data.

Student 4
Student 4

To remember: histograms have touching bars!

Teacher
Teacher

Exactly! They touch to show continuity. Remember the acronym H-T-C: Histogram-Touching-Continuity. Now let’s summarize.

Frequency Polygons

Unlock Audio Lesson

Signup and Enroll to the course for listening the Audio Lesson

Teacher
Teacher

Next up are frequency polygons, which relate closely to histograms. Who can explain what a frequency polygon is?

Student 1
Student 1

Is it like a line graph that uses the midpoints of histogram bars?

Teacher
Teacher

Exactly, Student_1! In a frequency polygon, we connect the midpoints of the tops of the bars in a histogram with straight lines. This gives us a clear visual representation of the distribution.

Student 2
Student 2

So, we can use it for comparing two sets of data?

Teacher
Teacher

Yes! Frequency polygons are particularly useful when comparing distributions between two datasets, like test scores of two different classes. The touching lines illustrate where the data overlaps.

Student 3
Student 3

What if there's no data for the first or last class?

Teacher
Teacher

Good point! We can create an imaginary class with zero frequency before the first and after the last classes to maintain continuity. Remember, a well-constructed graph tells a complete story!

Student 4
Student 4

To summarize frequency polygons: connect midpoints and use zero-frequency classes for continuity.

Teacher
Teacher

Great summary! Remember, these tools help us visualize data, making it easier to understand at a glance. Let's move on to some exercises!

Introduction & Overview

Read a summary of the section's main ideas. Choose from Basic, Medium, or Detailed.

Quick Overview

This section discusses the importance of graphical representations of data, highlighting bar graphs, histograms, and frequency polygons.

Standard

In this section, we explore different methods of graphical data representation, including bar graphs, histograms of both uniform and varying widths, and frequency polygons. These visual tools are essential for simplifying complex data, allowing for easier comparison and interpretation.

Detailed

Detailed Summary

Graphical representation of data is crucial in statistics as it transforms complex numerical data into visual formats that are easier to analyze and interpret. In this section, we focus on three primary types of graphical representations:

  1. Bar Graphs: Used for categorical data, bar graphs consist of rectangular bars representing different categories with heights corresponding to their values. The width of the bars is uniform, and they are separated by spaces.
  2. Histograms: A type of bar graph that represents continuous numerical data. Histograms depict the frequency distribution of data across specified ranges (bins). Unlike bar graphs, histograms have no spaces between the bars because they represent continuous data intervals.
  3. Frequency Polygons: This graphical representation connects the midpoints of the top of bars in a histogram with lines. It's useful for showing changes over time or comparing different groups of data.

The significance of these representations lies in their ability to simplify data interpretation, facilitate comparisons, and enhance data visualization.

image-a1739f6c-9b9d-40e2-9b90-412b6100635c.png

Youtube Videos

Class 9th - Statistics - Graphical Representation of Data Histograms
Class 9th - Statistics - Graphical Representation of Data Histograms
Class 9th - Statistics - Graphical Representation of Data Frequency Polygon
Class 9th - Statistics - Graphical Representation of Data Frequency Polygon
Class 9th - Statistics - Graphical Representation of Data Bar Graphs
Class 9th - Statistics - Graphical Representation of Data Bar Graphs
Graphical Representation of Data (Bar Graph) | Class 9 Maths Chapter 14
Graphical Representation of Data (Bar Graph) | Class 9 Maths Chapter 14
STATISTICS ONE SHOT | Full Chapter | Class 9 Maths | Chapter 14
STATISTICS ONE SHOT | Full Chapter | Class 9 Maths | Chapter 14
Maths Statistics part 12 (Numerical: Graphical Representation of Data) CBSE class 9 Mathematics IX
Maths Statistics part 12 (Numerical: Graphical Representation of Data) CBSE class 9 Mathematics IX
STATISTICS L-2 [Graphical Representation of Data] CBSE Class 9 Maths Chapter 14 (Term 1) | Vedantu
STATISTICS L-2 [Graphical Representation of Data] CBSE Class 9 Maths Chapter 14 (Term 1) | Vedantu
Statistics | Graphical Representation of Data Bar Graph | English Medium | Class 9 Term 1 |AT
Statistics | Graphical Representation of Data Bar Graph | English Medium | Class 9 Term 1 |AT
Solving Class 9 Maths: EX 1.2 (Incredible Techniques!)Ex 1.2 Q no 2( I to v)| Ali pasha
Solving Class 9 Maths: EX 1.2 (Incredible Techniques!)Ex 1.2 Q no 2( I to v)| Ali pasha
Graphical Representation of Data (Statistics) 🌟 Class 9 Maths Chapter 14 🌟 CBSE, NCERT 🎯
Graphical Representation of Data (Statistics) 🌟 Class 9 Maths Chapter 14 🌟 CBSE, NCERT 🎯

Audio Book

Dive deep into the subject with an immersive audiobook experience.

Introduction to Graphical Representation

Unlock Audio Book

Signup and Enroll to the course for listening the Audio Book

The representation of data by tables has already been discussed. Now let us turn our attention to another representation of data, i.e., the graphical representation. It is well said that one picture is better than a thousand words. Usually comparisons among the individual items are best shown by means of graphs. The representation then becomes easier to understand than the actual data. We shall study the following graphical representations in this section.

(A) Bar graphs
(B) Histograms of uniform width, and of varying widths
(C) Frequency polygons

Detailed Explanation

This chunk introduces the concept of graphical representation of data. Unlike tables, which show data in rows and columns, graphical representation uses visual forms like graphs to make data easier to understand. It supports the idea that visual depictions can convey information more effectively than numerical data alone. The section will cover different types of graphs: bar graphs, histograms, and frequency polygons.

Examples & Analogies

Imagine trying to show the number of books read by your friends in a month: if you list out each friend's name with numbers in a table, it might be hard to see who read the most. However, if you draw a bar graph where each bar represents a friend, you'll instantly see which bars are taller, indicating who read more books. This visual comparison is much quicker and easily understandable.

Understanding Bar Graphs

Unlock Audio Book

Signup and Enroll to the course for listening the Audio Book

(A) Bar Graphs

In earlier classes, you have already studied and constructed bar graphs. Here we shall discuss them through a more formal approach. Recall that a bar graph is a pictorial representation of data in which usually bars of uniform width are drawn with equal spacing between them on one axis (say, the x-axis), depicting the variable. The values of the variable are shown on the other axis (say, the y-axis) and the heights of the bars depend on the values of the variable.

Detailed Explanation

A bar graph visually represents data using bars, where the length or height of each bar corresponds to the size of the data it represents. Each bar has a uniform width and standard spacing. The x-axis typically shows the categories being compared, and the y-axis shows the values. For example, if you have data about students' favorite fruits, the x-axis would list the fruits, while the y-axis would represent the number of students who chose each fruit.

Examples & Analogies

Think about a carnival where different games have different numbers of players. A bar graph could display each game like a frog jumping contest or a ring toss on the x-axis, and how many players participated on the y-axis. This way, it would be clear at a glance which game was the most popular!

Example of Bar Graph Construction

Unlock Audio Book

Signup and Enroll to the course for listening the Audio Book

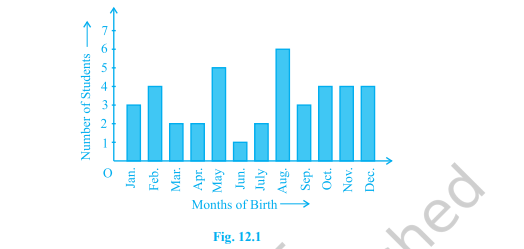
Example 1: In a particular section of Class IX, 40 students were asked about the months of their birth and the following graph was prepared for the data so obtained: [Bar Graph Reference]

Observe the bar graph given above and answer the following questions:
(i) How many students were born in the month of November?
(ii) In which month were the maximum number of students born?

Solution: Note that the variable here is the ‘month of birth’, and the value of the variable is the ‘Number of students born’. (i) 4 students were born in the month of November. (ii) The Maximum number of students were born in the month of August.

Detailed Explanation

This example illustrates how a bar graph can be used to analyze data. In this case, the data shows the birth months of students. By looking at the heights of the bars in the graph, one can quickly see how many students were born in each month and identify both the months with the fewest and most births.

Examples & Analogies

Imagine you’re at a birthday party and you want to know when most of your friends were born. By using a bar graph, you can visualize how many friends have birthdays in each month just with a glance, easily spotting the busiest birthday month at the party.

Constructing a Bar Graph - An Activity

Unlock Audio Book

Signup and Enroll to the course for listening the Audio Book

Let us now recall how a bar graph is constructed by considering the following example. Example 2: A family with a monthly income of ₹20,000 had planned the following expenditures per month under various heads: [Expenditure Data Table]

Draw a bar graph for the data above.

Detailed Explanation

This activity leads students through the steps of constructing a bar graph. The expenditure data provides various categories (like Grocery, Rent, etc.), and students learn how to represent these categories visually. They begin by labeling the axes, choosing a scale, and plotting the bars according to the expenditure values indicated.

Examples & Analogies

Consider budgeting your pocket money for different activities like games, books, or snacks. If you make a bar graph showing how much money you plan to spend on each, it will help you visualize your budget effectively and make adjustments as needed.

Understanding Histograms

Unlock Audio Book

Signup and Enroll to the course for listening the Audio Book

(B) Histogram
This is a form of representation like the bar graph, but it is used for continuous class intervals. For instance, consider the frequency distribution Table 12.2, representing the weights of 36 students of a class: [Weight Data Table].

Detailed Explanation

A histogram is similar to a bar graph but specifically used for continuous data, where the data is grouped into ranges (or intervals) rather than distinct categories. The heights of the bars correspond to the frequency of data points within each interval, allowing for quick visual interpretation of the distribution of values.

Examples & Analogies

Imagine measuring the heights of a class of students; instead of writing down every height, you group them into ranges (like 150-160 cm, 160-170 cm). A histogram would show how many students fall into each height range, giving you a clear picture of the height distribution in your class.

Histogram with Varying Widths

Unlock Audio Book

Signup and Enroll to the course for listening the Audio Book

Now, consider a situation different from the one above. Example 3: A teacher wanted to analyse the performance of two sections of students in a mathematics test of 100 marks. [Test Score Data Table].

Detailed Explanation

In this example, you see how to create a histogram when the intervals are not of uniform width. The key here is to ensure the areas of the bars represent the frequencies accurately even if the widths vary. This is vital to avoid any misleading interpretations of the data.

Examples & Analogies

Think about measuring the time taken by runners over a race, where some runners take varying times to finish different segments. If you represent that on a graph, ensuring the length of each segment accurately represents the number of runners requires careful calculations, similar to the varying widths of intervals in this histogram.

Introduction to Frequency Polygons

Unlock Audio Book

Signup and Enroll to the course for listening the Audio Book

(C) Frequency Polygon
There is yet another visual way of representing quantitative data and its frequencies. This is a polygon. To see what we mean, consider the histogram represented by Fig. 12.3. Let us join the mid-points of the upper sides of the adjacent rectangles of this histogram by means of line segments.

Detailed Explanation

A frequency polygon is formed by connecting the midpoints of the tops of the bars in a histogram with straight lines. This creates a shape that effectively visualizes how data values are distributed, similar to how the histogram does but often providing a clearer indication of trends and patterns.

Examples & Analogies

Think of a frequency polygon like the path of a roller coaster in an amusement park, where each peak and trough represents the data (like the number of students) at various intervals (like scores in a test). Just as the highs and lows give you an exciting overview of the ride, the peaks and valleys of a frequency polygon can provide an exciting picture of data trends.

Constructing a Frequency Polygon

Unlock Audio Book

Signup and Enroll to the course for listening the Audio Book

Now, the question arises: how do we complete the polygon when there is no class preceding the first class? Let us consider such a situation. Example 4: Consider the marks, out of 100, obtained by 51 students of a class in a test. [Marks Data Table].

Detailed Explanation

When creating a frequency polygon where there's no class interval before the first, we extend the horizontal axis backward to create an imaginary class with zero frequency. This practice ensures that the polygon accurately reflects the overall shape and area relative to the original histogram.

Examples & Analogies

Imagine you're drawing a line on a graph that represents temperatures over a day. If a certain hour of the day had no data recorded, you can still put a point at that hour to show that the temperature there was zero, maintaining the flow of your graph, just like how we manage continuity for our frequency polygons.

Definitions & Key Concepts

Learn essential terms and foundational ideas that form the basis of the topic.

Key Concepts

  • Bar Graph: A visual representation for categorical data with rectangular bars.

  • Histogram: A visual for continuous data with adjacent bars.

  • Frequency Polygon: A line graph connecting midpoints of histogram bars.

Examples & Real-Life Applications

See how the concepts apply in real-world scenarios to understand their practical implications.

Examples

  • {'example': 'Draw a bar graph for the following data:\n\n| Fruit | Votes |\n|-------------|-------|\n| Apples | 20 |\n| Oranges | 30 |\n| Bananas | 25 |', 'solution': 'The heights of the bars would be 20, 30, and 25 respectively for Apples, Oranges, and Bananas.'}

  • {'example': 'Draw a histogram for the following data:\n\n| Weight Interval (kg) | Number of Students |\n|----------------------|--------------------|\n| 30.5 - 35.5 | 9 |\n| 35.5 - 40.5 | 6 |\n| 40.5 - 45.5 | 15 |', 'solution': 'For each interval, draw bars of widths corresponding to class intervals with heights relating to frequencies.'}

Memory Aids

Use mnemonics, acronyms, or visual cues to help remember key information more easily.

🎵 Rhymes Time

  • Bars are tall, gaps are none, histograms show continuous fun!

📖 Fascinating Stories

  • A teacher explains to students how a fruit market uses bar graphs to display fruits sold, ensuring learning is sweet like apples and oranges they sell.

🧠 Other Memory Gems

  • B-H-F: Bar graphs show Categories, Histograms show Intervals, Frequency Polygons show Connections.

🎯 Super Acronyms

H-T-C

  • Remember Histograms Touch for continuity.

Flash Cards

Review key concepts with flashcards.

Glossary of Terms

Review the Definitions for terms.

  • Term: Bar Graph

    Definition:

    A graph using bars to represent categorical data.

  • Term: Histogram

    Definition:

    A graphical representation of data using bars for continuous data, with no gaps between bars.

  • Term: Frequency Polygon

    Definition:

    A line graph created by connecting midpoints of the frequencies represented by the bars in a histogram.