12.1.B - Histogram
Enroll to start learning
You’ve not yet enrolled in this course. Please enroll for free to listen to audio lessons, classroom podcasts and take practice test.
Interactive Audio Lesson
Listen to a student-teacher conversation explaining the topic in a relatable way.
Introduction to Histograms
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

Today, we are going to explore histograms. Can anyone tell me how a histogram is different from a bar graph?

A bar graph shows categories, while a histogram is for continuous data, right?

Exactly! In histograms, the data is continuous, depicted without gaps. This means each bar touches the next. Remember, histograms represent frequencies of continuous intervals.

So, if we have weights, we would group them and represent those groups on a histogram?

Exactly right! Let’s dive deeper into the steps for constructing a histogram.
Constructing a Histogram
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

To construct a histogram, you start by defining your class intervals. Who can tell me how we might choose those intervals?

We would look at the range of data and create intervals of equal widths, right?

Correct! However, in some cases, like test scores, the widths can vary. We’ll adjust later by modifying rectangle heights accordingly.

And what about the scales on the axes?

Good question! The horizontal axis represents the class intervals while the vertical axis represents frequency. Scaling correctly is key for accurate representation.
Example of Histogram Construction
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

Let’s apply what we learned. Here is a frequency table showing the weights of students. How would we begin to construct the histogram?

We need to plot each weight range on the horizontal axis based on the classes.

Exactly! Then, plot frequencies on the vertical axis. Let’s say our frequency for 30.5 - 35.5 kg is 9; we’ll draw the corresponding rectangle.

Wait, what if the frequency doesn’t fit? Do we adjust the height?

If the widths vary, yes! It’s important to ensure areas are proportional to frequencies.
Analyzing Histograms
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

Now that we have created our histogram, how do we interpret it?

We can see where most students fit weight-wise and identify ranges with more frequency.

Great insight! Are there any limitations or common mistakes we should be aware of?

If the intervals aren’t uniform, it might misrepresent the frequency visually.

Exactly! Always ensure your areas correspond to frequency.
Introduction & Overview
Read summaries of the section's main ideas at different levels of detail.
Quick Overview
Standard
This section introduces histograms, explaining how they differ from bar graphs by representing continuous data without gaps. It outlines the steps to construct histograms for grouped frequency distributions, emphasizes the importance of area proportionality to frequency, and addresses common pitfalls when dealing with varying class widths.
Detailed
Detailed Summary
In this section, we delve into histograms as a vital graphical tool for representing continuous data. Unlike bar graphs, histograms display continuous class intervals without gaps, emphasizing the distribution of a variable.
Key Points Covered:
- Definition: A histogram is a visual representation of frequency distributions where the data is displayed in contiguous bars, each representing a class interval.
- Construction Steps: To construct a histogram, one must:
- Define the class intervals.
- Determine the frequency for each class interval.
- Choose an appropriate scale for the horizontal and vertical axes.
- Avoid gaps by ensuring rectangular bars touch.
- (For varying widths) Adjust areas of rectangles to align with frequencies.
- Examples & Applications: The section includes examples using class frequencies from student weights and testing scores, illustrating practical applications of histograms in educational assessments.
- Misleading Histograms: It highlights issues with improperly constructed histograms, particularly when class widths vary. The area of rectangles must correspond to frequencies to ensure accurate representation.
- Connection to Other Graphical Representations: Histograms lead into discussions on frequency polygons, illustrating how they can complement histograms by connecting mid-point frequencies visually.
In summary, histograms are crucial for interpreting data distributions and provide a foundational understanding necessary for further statistical analysis.
Youtube Videos










Audio Book
Dive deep into the subject with an immersive audiobook experience.
Basic Concept of a Histogram
Chapter 1 of 6
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
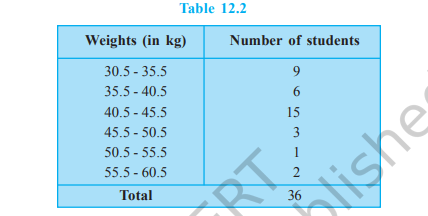
A histogram is a form of representation like the bar graph, but it is used for continuous class intervals. For instance, consider the frequency distribution Table 12.2, representing the weights of 36 students of a class:
Detailed Explanation
A histogram is similar to a bar graph but is specifically designed for continuous data, where the data falls into ranges or intervals. It visually displays how many data points fall within each range (or class interval). In the example provided, we will be looking at the weights of 36 students.
Examples & Analogies
Imagine measuring the heights of children at a school. Instead of knowing the exact height of each child, we might be interested in how many children fall into certain height ranges, like 4-5 feet, 5-6 feet, etc. A histogram helps us quickly see how many children fall into each height range.
Steps to Draw a Histogram
Chapter 2 of 6
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
(i) We represent the weights on the horizontal axis on a suitable scale. We can choose the scale as 1 cm = 5 kg. Also, since the first class interval is starting from 30.5 and not zero, we show it on the graph by marking a kink or a break on the axis.
Detailed Explanation
To create a histogram, we start by plotting our intervals along the horizontal axis (x-axis). We choose a scale that allows us to represent our data clearly. If our first class interval starts at 30.5 kg, we need to indicate that this is not starting from zero by adding a break in the axis. This ensures our visualization accurately reflects the data.
Examples & Analogies
Think about a ramp that starts at the ground level (zero). If our first data point starts a little above the ground, we need to create a clear break showing that the ramp starts higher and doesn't touch the ground.
Representing Frequencies
Chapter 3 of 6
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
(ii) We represent the number of students (frequency) on the vertical axis on a suitable scale. Since the maximum frequency is 15, we need to choose the scale to accommodate this maximum frequency.
Detailed Explanation
Next, we plot the frequencies of our intervals along the vertical axis (y-axis). We need to ensure that our scale allows us to visualize the highest grouping clearly. If the highest number of students in any interval is 15, our y-axis scale must be enough to represent at least this number.
Examples & Analogies
It's like measuring how many students scored above a certain grade. If the highest score is 100 in a test and you decided to plot it, you should ensure your y-axis reaches higher than 100 so everyone can see the scores clearly.
Drawing Rectangles for Class Intervals
Chapter 4 of 6
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
(iii) We now draw rectangles (or rectangular bars) of width equal to the class-size and lengths according to the frequencies of the corresponding class intervals.
Detailed Explanation
Now it’s time to draw the histogram. Each interval will be represented by a rectangle where the width corresponds to the class size (e.g., from 30.5 to 35.5 is one interval), and the height will reflect the number of students (frequency) in that range. Therefore, if we have 9 students in the 30.5-35.5 kg interval, we draw a rectangle above that bar extending to 9 on the vertical scale.
Examples & Analogies
Consider building a wall of different heights to represent how many people fit into different height groups. If 9 people are between 30.5 and 35.5 kg, you would build a wall that rises 9 bricks high at that position.
Characteristics of a Histogram
Chapter 5 of 6
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
Observe that since there are no gaps in between consecutive rectangles, the resultant graph appears like a solid figure. This is called a histogram, which is a graphical representation of a grouped frequency distribution with continuous classes.
Detailed Explanation
The key feature of a histogram is that there are no gaps between adjacent rectangles. This solid appearance signifies that the data are continuous, meaning they flow into each other without interruption. Each rectangle represents a range of data points, and the height is proportional to the frequency of those points.
Examples & Analogies
Think of a water slide at an amusement park. The slide is continuous; you go from one section to another without any breaks or gaps in between. Just like that, a histogram allows for a smooth transition between values, showing how data flows through different intervals.
Importance of Width in Histogram
Chapter 6 of 6
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
Also, unlike a bar graph, the width of the bar plays a significant role in its construction. Here, in fact, areas of the rectangles erected are proportional to the corresponding frequencies.
Detailed Explanation
In a histogram, the width of each rectangle can impact how the frequency is represented, especially when intervals vary in size. It's important to ensure that the area of each rectangle is proportional to the frequency of the data it represents. In simpler terms, wider intervals should have corresponding heights adjusted accordingly to ensure accurate representation.
Examples & Analogies
Imagine a garden with flower beds of various sizes. If one bed is much wider, but has fewer flowers than a narrower bed, just counting the number of flowers may give a misleading picture. The size of each bed (or bar) matters to understand how many flowers are truly there in comparison to each other.
Key Concepts
-
Definition of Histogram: A visual tool to represent frequency distribution for continuous data.
-
Construction Steps: Involves choosing intervals, scaling axes, and ensuring correct representation of frequencies.
Examples & Applications
{'example': 'Example 1: Construct a histogram for the given student weight data.', 'solution': 'The histogram representation involves plotting weights on the x-axis and the corresponding frequencies on the y-axis, ensuring bars connect.'}
Memory Aids
Interactive tools to help you remember key concepts
Rhymes
A histogram's bars rise high, showing data as they lie.
Stories
Imagine you are a baker. Each loaf of bread represents a frequency of different weights. The more bread of one weight, the taller the stack, forming a histogram that tells you about all the loaves you have!
Memory Tools
H.I.S.: Histogram Includes Spaced bars (no gaps!)
Acronyms
HISTO
Histogram Illustrates Statistical Trends Over.
Flash Cards
Glossary
A graphical representation of frequency distributions for continuous class intervals, visualized through contiguous bars.
The number of occurrences of a particular value or range of values in a dataset.
A range of values in a frequency distribution that groups data points.
Reference links
Supplementary resources to enhance your learning experience.
