Professional Courses
Industry-relevant training in Business, Technology, and Design to help professionals and graduates upskill for real-world careers.
Categories
Interactive Games
Fun, engaging games to boost memory, math fluency, typing speed, and English skillsβperfect for learners of all ages.
Typing
Memory
Math
English Adventures
Knowledge
Enroll to start learning
Youβve not yet enrolled in this course. Please enroll for free to listen to audio lessons, classroom podcasts and take mock test.
Interactive Audio Lesson
Listen to a student-teacher conversation explaining the topic in a relatable way.
Introduction to Triangle Congruence
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson
Today we will explore how we determine if two triangles are congruent. Can anyone remind me why congruence is important?
It's important because it helps us know that two shapes are identical in size and shape.
Exactly! And what do you think are the criteria we can use to verify triangle congruence?
I remember something about sides and angles. Maybe Side-Angle-Side?
What about Angle-Side-Angle too?
Great! Those are indeed two of the criteria. Let's dive into them now starting with the SAS criterion.
Side-Angle-Side (SAS) Criterion
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson
The SAS criterion states that if two sides and the included angle are equal, the triangles are congruent. Can someone give me an example?
If triangle ABC has sides of lengths 5 cm and 7 cm and included angle 60Β°, and triangle DEF has the same measurements, they would be congruent!
Nice example! Remember, the angle we consider must always be between the two sides we compare. Can anyone think of a way to remember these criteria?
Maybe we could say 'SAS = Side-Angle-Side'?
That's a solid memory aid! Keep that in mind as we explore the next criterion.
Angle-Side-Angle (ASA) Criterion
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson
Moving on to ASA: two angles and the included side are equal. Can someone explain how this might work in practice?
If I have triangle GHI with angles of 30Β° and 40Β° and a side of 10 cm, then triangle JKL with the same measurements must be congruent.
Exactly! And it's crucial that the side is between the two angles. Now, why do you think we should learn multiple criteria?
It gives us flexible options to prove congruence depending on the given information!
Correct! Let's remember that having multiple methods aids problem-solving.
Angle-Angle-Side (AAS) Criterion
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson
Now letβs discuss AAS. Can anyone summarize what this involves?
Two angles and a side that is not included means the triangles are congruent?
That's correct! AAS is useful when we know two angles and one non-included side. Can anyone think of how this helps us in real applications?
In architecture, you might need to ensure that certain angles are the same to make parts fit together!
Exactly! Understanding congruence has many practical applications in design and construction.
Side-Side-Side (SSS) and RHS Criteria
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson
Finally, we have the SSS criterion, which states that if all three sides of one triangle are equal to another's, they are congruent. Why do we compare all sides?
Because it ensures the shape and size are identical!
Correct! And what about the RHS criterion for right triangles?
If the hypotenuse and one side of one right triangle are equal to those in another right triangle, the two are congruent!
Well done! Remember these criteria as they will continuously appear in geometry. Let's summarize what we've learned today.
Today, we reviewed congruence in triangles, focusing on the SAS, ASA, AAS, SSS, and RHS criteria. Each allows us to prove that two triangles are congruent based on different known elements. Understanding these can aid in both problem solving and real-world applications.
Introduction & Overview
Read a summary of the section's main ideas. Choose from Basic, Medium, or Detailed.
Quick Overview
Standard
In this section, we recap the specific criteria for triangle congruence, including SAS, ASA, AAS, SSS, and RHS rules. Examples are provided to illustrate how these criteria apply, and exercises challenge the understanding of congruence in triangles.
Detailed
Detailed Summary
In the study of triangles, congruence is a fundamental concept that signifies two figures having the same shape and size. This section revisits the criteria for establishing the congruence of triangles, which include:
- Side-Angle-Side (SAS) Criterion: This states that two triangles are congruent if two sides and the included angle of one triangle are equal to the two sides and the included angle of the other triangle.
- Angle-Side-Angle (ASA) Criterion: Two triangles are congruent if two angles and the included side of one triangle are equal to the two angles and the included side of the other triangle.
- Angle-Angle-Side (AAS) Criterion: This criterion holds true when two angles and a non-included side of one triangle are equal to the corresponding parts of another triangle.
- Side-Side-Side (SSS) Criterion: This implies that if all three sides of one triangle are equal to all three sides of another triangle, the triangles are congruent.
- Right angle-Hypotenuse-Side (RHS) Criterion: Applicable in right triangles, this declares that if the hypotenuse and one side of one right triangle are equal to the hypotenuse and one side of another right triangle, then the two triangles are congruent.
Examples in this section provide practical applications of these criteria, thereby enhancing understanding. Exercises are presented to reinforce these concepts through problem-solving and verification of congruence in various scenarios.
Youtube Videos
Audio Book
Dive deep into the subject with an immersive audiobook experience.
Introduction to Criteria for Congruence
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
In earlier classes, you have learnt four criteria for congruence of triangles. Let us recall them.
Draw two triangles with one side 3 cm. Are these triangles congruent? Observe that they are not congruent (see Fig. 7.5).
Detailed Explanation
This introduction sets the stage for understanding what it means for triangles to be congruent. Congruence implies that two triangles have the same shape and size, with corresponding sides and angles that are equal. The given example of drawing two triangles with one side each of 3 cm emphasizes that having just one side equal is not enough for the triangles to be congruent, as additional sides and angles must also be considered.
Examples & Analogies
Think of two identical shoes; if one shoe is missing a key detail, such as the correct size or built, even if they feel similar at first glance, they will not fit right. Similarly, for triangles, it's not enough to have one measurement match; all relevant features must align.
Exploring the Criteria Further
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Now, draw two triangles with one side 4 cm and one angle 50Β° (see Fig. 7.6). Are they congruent? See that these two triangles are not congruent. Repeat this activity with some more pairs of triangles.
Detailed Explanation
Here, we observe another scenario where only one side and one angle are equal. The emphasis is on testing the congruence by drawing and comparing various pairs of triangles. The conclusion drawn from these activities reinforces that knowing just a side and an angle does not guarantee congruence, as the overall configuration of the triangles could differ.
Examples & Analogies
Imagine building a model airplane using different parts. Even if you use one identical wing and a similar engine, the alignment of all components must be precise for the airplane to fly correctly. Just like that, triangles must maintain alignment across all sides and angles to be congruent.
The SAS Congruence Rule
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
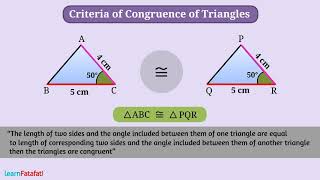
In Fig 7.7, BC = QR, β B = β Q and also, AB = PQ. Now, what can you say about congruence of βABC and βPQR? Recall from your earlier classes that, in this case, the two triangles are congruent. This is the first criterion for congruence of triangles.
Axiom 7.1 (SAS congruence rule): Two triangles are congruent if two sides and the included angle of one triangle are equal to the two sides and the included angle of the other triangle.
Detailed Explanation
This segment presents the SAS (Side-Angle-Side) congruence rule. Here, we see how having two sides and the angle between them equal for two triangles ensures their overall congruence. This criterion is a foundational tool in geometry. Students are encouraged to verify this in practice through constructions of triangles based on these conditions.
Examples & Analogies
Consider two bridges made with the same materials where the main beams are identical in length and the angle of the support is also the same. These structures will behave in the same manner under load due to their congruence, just as congruent triangles will have the same properties.
Explorations of SAS
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Let us now take some examples. Example 1: In Fig. 7.8, OA = OB and OD = OC. Show that (i) β AOD β β BOC and (ii) AD || BC.
Detailed Explanation
This example illustrates the practical application of the SAS rule. The equality of two sides and the included angle (formed by the line segments and transversals) leads to the conclusion that these two triangles are congruent, and hence the lines AD and BC are parallel. This showcases how geometric properties can derive from congruence.
Examples & Analogies
Think of constructing a skateboard ramp; if two sections are built with the same measurements for safety (length and angle), they ensure that skaters can use both parts seamlessly without risk, just like the parallel configuration derived from the triangles being congruent.
Further Criteria for Congruence
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Next, we learn about additional criteria. For instance, if two angles and one included side are equal, we have the ASA (Angle-Side-Angle) criterion. This can also be proved through constructing examples and observing congruence between triangles drawn with those conditions.
Detailed Explanation
The ASA criterion gives us another method to determine triangle congruence. By ensuring that two angles and the side between them are equal, we can conclude that the triangles must indeed be the same shape and size. This section promotes geometric dexterity by requiring students to visualize and construct triangles that fit the ASA model.
Examples & Analogies
Imagine two sailboats that are rigged identically with matching sails and a sail length. Because of these matching characteristics, they'll handle the same way in the wind. This is akin to how triangles behave under the criteria of ASA; their internal angles dictate their overall configuration and congruence.
Conclusion of Criteria for Congruence
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Finally, it is established that equality of three angles is not sufficient for congruence, reinforcing the need for incorporating at least one side equality into triangle properties.
Detailed Explanation
In closing, this section underlines a vital point about triangle congruence: having three angles equal does not guarantee congruence if the sides differ. Thus, students conclude with a robust understanding of how congruence functions across various criteria, and need to incorporate conditions judiciously.
Examples & Analogies
Consider two triangles you might sketch; although they could share angle shapes (like drawn protractors), if one triangle's base is longer than another, they won't be the same triangle. This practical visual can help students grasp why at least one side parameter is essential.
Definitions & Key Concepts
Learn essential terms and foundational ideas that form the basis of the topic.
Key Concepts
-
SAS Criterion: Two triangles are congruent if two sides and the included angle are equal.
-
ASA Criterion: Two triangles are congruent if two angles and the included side are equal.
-
AAS Criterion: Two triangles are congruent if two angles and a non-included side are equal.
-
SSS Criterion: Two triangles are congruent if all three sides are equal.
-
RHS Criterion: In right triangles, if the hypotenuse and one side are equal, then the triangles are congruent.
Examples & Real-Life Applications
See how the concepts apply in real-world scenarios to understand their practical implications.
Examples
-
Example applying SAS: If triangle ABC has sides AB = 5 cm, AC = 7 cm and angle A = 60Β°, it is congruent to triangle DEF with the same dimensions.
-
Example applying AAS: Triangle XYZ has angles of 50Β° and 60Β° with side XY = 10 cm. If triangle PQR has angles of 50Β° and 60Β° and side PQ = 10 cm, then they are congruent.
Memory Aids
Use mnemonics, acronyms, or visual cues to help remember key information more easily.
π΅ Rhymes Time
-
If two sides and the angle in between align, SAS will proclaim congruence just fine.
π Fascinating Stories
-
Once upon a time in Triangle Town, there were triangles with sides and angles. Whenever two angles and a side connected, they would celebrate their congruent friendship with AAS!
π§ Other Memory Gems
-
For remembering the criteria: 'SAS for sides and angle in the middle, ASA for angles and side, thatβs a riddle.'
π― Super Acronyms
You can use SSS, SAS, ASA and AAS, plus RHS for right triangles, thatβs how we measure the congruence gauge.
Flash Cards
Review key concepts with flashcards.