6.3.1 - Cube root through prime factorisation method
Enroll to start learning
You’ve not yet enrolled in this course. Please enroll for free to listen to audio lessons, classroom podcasts and take practice test.
Interactive Audio Lesson
Listen to a student-teacher conversation explaining the topic in a relatable way.
Introduction to Cube Roots
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

Today, we are going to explore how we can find the cube roots of numbers using prime factorisation. Can anyone tell me what a cube root is?

Isn't it the number that when multiplied by itself three times gives the original number?

Exactly, well done! For example, \(3^3 = 27\), so the cube root of 27 is 3. We represent this as \(\sqrt[3]{27} = 3\).

What if we have a bigger number, like 64?

Good question! We will show you how to find the cube root of larger numbers by breaking them down into their prime factors.

Could you give us an example?

Sure! Let's take 3375. I’ll show you how to factor it and find its cube root. So we find the prime factorisation first.

The factorisation is \(3375 = 3 \times 3 \times 3 \times 5 \times 5 \times 5 = 3^3 \times 5^3 = (3 \times 5)^3\). So what's the cube root of 3375?

That would be 15, right?

Correct! You're all doing great.
Examples of Cube Roots
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

Now that we have our first example, let’s try another number! What do you think is the cube root of 74088?

How do we start?

Let's begin by performing the prime factorisation of 74088. After the factorisation, we have \(74088 = 2^3 \times 3^3 \times 7^3\). Can someone tell me how to find the cube root from here?

Combine the factors from groups of three, right?

Yes! By combining those factors, we can rewrite the expression as \((2 \times 3 \times 7)^3\). Now what do we get?

The cube root of 74088 would be 42!

Absolutely correct! Remember the formula that links these concepts: \(\sqrt[3]{a^3} = a\) helps summarize what we just did.
Applying the Method
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

Let’s practice together. How about we find the cube root of 8000? What’s the first step?

We should factor 8000 into its prime factors!

That’s right! The factorization is \(8000 = 2^6 \times 5^3\). Now, can you tell me how to find the cube root?

We take the cube root of each factor? So \(\sqrt[3]{2^6} = 2^2\) and \(\sqrt[3]{5^3} = 5\).

Perfect! So what is the cube root of 8000?

That’s 20!

Excellent work, everyone! Have you noticed how the cube roots relate to their prime bases?

Yes, understanding it like that makes it easier!
Introduction & Overview
Read summaries of the section's main ideas at different levels of detail.
Quick Overview
Standard
The section explains the process of finding the cube root of a number through prime factorization, highlighting the importance of grouping factors in threes and providing examples for clarity. Additionally, it emphasizes the application of this method in determining cube roots of various numbers.
Detailed
Cube Root through Prime Factorisation Method
In this section, we learn how to find the cube root of a number using the prime factorisation method. The cube root is determined by expressing a number as the product of its prime factors and identifying how many times each factor is used in groups of three.
Two significant examples illustrated include:
1. Finding the cube root of 3375: The prime factorisation is done to express 3375 as \( 3375 = 3^3 \times 5^3 = (3 \times 5)^3 \). Hence, the cube root of 3375 is \( 3 \sqrt[3]{3375} = 15 \).
2. Finding the cube root of 74088: Following similar steps, we find \( 74088 = 2^3 \times 3^3 \times 7^3 = (2 \times 3 \times 7)^3 \), which leads to a cube root of \( \sqrt[3]{74088} = 42 \).
This method is significant as it not only offers a systematic approach to finding cube roots but also reinforces understanding of prime factorisation in mathematical terms.
Example 7: Find the cube root of 54000.
Solution: Prime factorisation of 54000 is
$$54000 = 2^3 \times 3^3 \times 5^3$$.
So,
$$\sqrt[3]{54000} = 2 \times 3 \times 5 = 30.$$
Similar Question:
Example 8: Find the cube root of 729000.
Solution: Prime factorisation of 729000 is
$$729000 = 3^6 \times 2^3 \times 5^3.$$
So,
$$\sqrt[3]{729000} = 3^2 \times 2 \times 5 = 45.$$
Youtube Videos
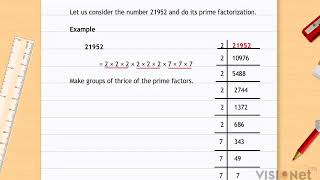







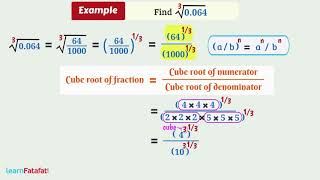
Audio Book
Dive deep into the subject with an immersive audiobook experience.
Understanding Cube Roots
Chapter 1 of 4
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
Consider 3375. We find its cube root by prime factorisation: 3375 = 3 × 3 × 3 × 5 × 5 × 5 = 33 × 53 = (3 × 5)3. Therefore, cube root of 3375 = 3 3375 = 3 × 5 = 15.
Detailed Explanation
To find the cube root of a number, we can use prime factorisation. A number can be expressed as a product of prime numbers. In this case, 3375 is broken down into its prime factors, where 3 and 5 appear three times each. Since each prime factor is raised to the power of 3, we take one of each for the cube root. Therefore, the cube root of 3375 is 15, calculated as the product of the prime factors that appear to the first power.
Examples & Analogies
Think of a cube of sugar. If you have a cube with a volume of 3375 cm³, using the prime factorisation method is like figuring out how many small sugar cubes of side 15 cm can fit in. Since each small cube has a volume of 15 × 15 × 15, we can conclude that there are enough small cubes to perfectly form the larger one.
Finding Another Cube Root
Chapter 2 of 4
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
Similarly, to find \( \sqrt[3]{74088} \), we have, 74088 = 2 × 2 × 2 × 3 × 3 × 3 × 7 × 7 × 7 = 2³ × 3³ × 7³ = (2 × 3 × 7)³. Therefore, \( \sqrt[3]{74088} = 2 × 3 × 7 = 42. \)
Detailed Explanation
To find the cube root of 74088, we again use prime factorisation. Here, 74088 can be factored into three groups of the primes 2, 3, and 7, all raised to the power of 3. By taking the product of these base factors, we get 42, which is the cube root of 74088.
Examples & Analogies
Imagine you have a fruit basket filled with different types of fruit: apples, bananas, and mangoes, where each type appears equally because of the factory process. Each group of three fruits represents the cube root, and if you find out the total count of fruit types grouped into equal sets, this is akin to finding the cube root of 74088, revealing that you can create 42 smaller baskets.
Example of Finding a Cube Root
Chapter 3 of 4
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
Example 6: Find the cube root of 8000. Solution: Prime factorisation of 8000 is 2 × 2 × 2 × 2 × 2 × 2 × 5 × 5 × 5. So, \( \sqrt[3]{8000} = 2 × 2 × 5 = 20. \)
Detailed Explanation
To find the cube root of 8000, we factor it down to its prime components. The prime factorisation shows that there are six 2's and three 5's. Grouping these factors in sets of three to simplify, we take two 2's and one 5 to determine that the cube root is 20.
Examples & Analogies
Consider a large bag of flour weighing 8000 grams. If we want to form equal cube-shaped portions from this bag, we would find the number of portions that can be created in the shape of 20-gram cubes. Hence, the cube's side of 20 grams allows us to efficiently utilize the entire bag.
Another Example: Cube Root of 13824
Chapter 4 of 4
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
Example 7: Find the cube root of 13824 by prime factorisation method. Solution: 13824 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 3 × 3 × 3 = 2³ × 2³ × 3³. Therefore, \( \sqrt[3]{13824} = 2 × 2 × 2 × 3 = 24. \)
Detailed Explanation
In finding the cube root of 13824, we illustrate the process of prime factorisation by breaking it down into prime factors: we have a total of nine 2's and three 3's. By forming groups of three, we deduce that the cube root is 24, representing the side length of the cube.
Examples & Analogies
Imagine you are making stacks of boxes, where each box fits perfectly in the larger cube container. If you want to create stacks based on the number 13824, similar to layering boxes, the cube root demonstrates that 24 boxes neatly fill up the larger area without any empty space.
Key Concepts
-
Cube Root: The number which when multiplied by itself three times gives the original number.
-
Prime Factorization: This process involves breaking down a number into its prime factors.
-
Perfect Cube: A number that can be expressed as the cube of an integer.
-
Prime Factor Grouping: To find the cube root using prime factors, arrange factors in groups of three.
Examples & Applications
Example 1: Finding the cube root of 3375 gives 15.
Example 2: Finding the cube root of 74088 gives 42.
Memory Aids
Interactive tools to help you remember key concepts
Rhymes
To find a cube with delight, group the factors tight. Three is the key, it's a simple sight.
Acronyms
C.U.B.E – Calculate, Understand, Break down, Extract the root!
Memory Tools
For cube roots, remember: Group triplets, find the root, that's the best route!
Stories
Once upon a time, in a land of numbers, the king wanted to find the hidden secret of every cube. His wise advisor told him, 'Cube root is the key! Just group the factors in threes!' So they explored the kingdom of primes and unveiled the treasures of perfect cubes!
Flash Cards
Glossary
- Cube Root
A number which when multiplied by itself three times gives the original number.
- Prime Factorization
Breaking down a number into its basic building blocks, which are prime numbers.
- Perfect Cube
A number that can be expressed as the cube of an integer.
- HardyRamanujan Number
A number that can be expressed as the sum of two cubes in more than one way.
Reference links
Supplementary resources to enhance your learning experience.
