8.4.1 - Multiplying a monomial by a binomial
Enroll to start learning
You’ve not yet enrolled in this course. Please enroll for free to listen to audio lessons, classroom podcasts and take practice test.
Interactive Audio Lesson
Listen to a student-teacher conversation explaining the topic in a relatable way.
Understanding Monomials and Binomials
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

Today we're going to talk about monomials and binomials. Who can tell me what a monomial is?

Is it a math expression with only one term?

Exactly! And a binomial, can someone explain that?

A binomial has two terms, right?

Correct! For example, `5x + 2` is a binomial. Remember, when multiplying a monomial by a binomial, we use the distributive law to help us.

How does that work exactly?

Great question! Let's use `3x` and the binomial `5y + 2`. We distribute and multiply each term. It looks like this: `3x × (5y + 2) = (3x × 5y) + (3x × 2)`, which simplifies to `15xy + 6x`. Remember the acronym 'DISTRIBUTE' to aid your memory: 'Distribute Each Term'.

So we just multiply like we would with numbers?

Exactly! Let's summarize: we multiply each part of the binomial by the monomial. Who can give me another example?
Applying the Distributive Property
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

Let's dive into applying the distributive property! Who can remind us what it means?

It means to multiply each term in the parentheses by what’s outside.

Exactly! For instance, with `-2a × (3b - 4)`, we get `-2a × 3b + (-2a) × (-4)`. What do we get?

That would be `-6ab + 8a`.

Well done! Notice how two negatives make a positive. Can anyone think of why we might reorder terms?

To simplify calculations! If we multiply `(3b - 4) × -2a`, we'd end with the same terms but possibly different signs!

Great observation! This property allows flexibility in computation.

Can we also multiply a binomial with another binomial using the same rules?

Yes! As we progress, we'll practice that, reinforcing our understanding of these laws.
Working with Negative Coefficients
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

Next, let’s tackle expressions with negative numbers. For example, what happens with `-3x(5y + 2)`?

You would apply the same principle, right?

Absolutely! We would do `(-3x) × 5y + (-3x) × 2`, resulting in `-15xy - 6x`. Remember: 'Negative times Positive equals Negative; Negative times Negative equals Positive' – that’s a useful saying!

Could we rewrite it as `(-3x)(5y) + (-3x)(2)` to see it clearer?

Yes! It helps visualize the operation. Consistent practice ensures you understand these transformations.

Can you summarize again how to notice when signs change?

Sure! Just keep in mind the rules of signs when multiplying—this will guide you through.
Introduction & Overview
Read summaries of the section's main ideas at different levels of detail.
Quick Overview
Standard
It provides a method for multiplying monomials and binomials, particularly through the distributive property, ensuring students grasp the structured approach of multiplying each term in the binomial by the monomial and combining like terms.
Detailed
Multiplying a Monomial by a Binomial
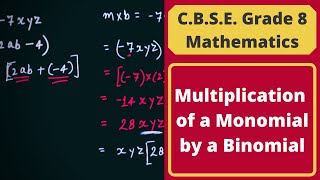
In this section, we explore the multiplication of a monomial by a binomial, emphasizing the use of the distributive law to facilitate the process. A monomial is defined as an expression containing only one term, while a binomial is an expression that contains two terms. The distributive property allows us to expand expressions effectively.
To multiply a monomial, such as 3x, by a binomial, like 5y + 2, we use the formula:
3x × (5y + 2) = (3x × 5y) + (3x × 2).
Following the multiplication, we combine the results: 15xy + 6x. The section further illustrates that the order of multiplication does not affect the outcome, as shown by the example that reverses the positions of the monomial and binomial. This foundational concept is critical for understanding more complex polynomial operations later in algebra.
Youtube Videos








Key Concepts
-
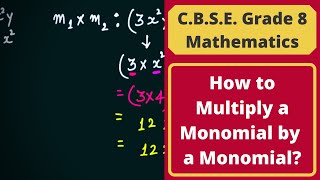
Distributive Law: Necessary rule for multiplying monomials with polynomials.
-
Term Multiplication: Each term in the binomial or polynomial must be multiplied by the monomial.
Examples & Applications
Example 1: Multiply 3x by the binomial 5y + 2 to get 15xy + 6x.
Example 2: Multiply -2a by 3b - 4 to receive -6ab + 8a.
Memory Aids
Interactive tools to help you remember key concepts
Rhymes
To multiply a mono and a bino,/ Just distribute as you go,/ Don’t forget to combine the like,/ Or your answer may take a hike.
Stories
Once there was a clever little monomial named 3x who had a special friendship with the binomial 5y + 2. They loved to party, so every time they met, they multiplied their terms and had fun!
Memory Tools
Daisy Eats Sweet Bananas - for 'Distribute Each term in the Sum of the Binomial'.
Acronyms
MATH - Monomial and a Binomial Together Harmoniously.
Flash Cards
Glossary
- Monomial
An algebraic expression consisting of a single term, such as
3x.
- Binomial
An algebraic expression containing two terms, such as
5y + 2.
- Distributive Law
A property that states a(b + c) = ab + ac, used for distributing multiplication over addition.
- Coefficient
A numerical factor in a term, such as
3in3x.
Reference links
Supplementary resources to enhance your learning experience.
