Professional Courses
Industry-relevant training in Business, Technology, and Design to help professionals and graduates upskill for real-world careers.

Categories
Interactive Games
Fun, engaging games to boost memory, math fluency, typing speed, and English skills—perfect for learners of all ages.

Typing
Memory
Math
English Adventures
Knowledge
Enroll to start learning
You’ve not yet enrolled in this course. Please enroll for free to listen to audio lessons, classroom podcasts and take mock test.
Interactive Audio Lesson
Listen to a student-teacher conversation explaining the topic in a relatable way.
Introduction to Monomials and Trinomials
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson

Good morning, class! Today we will learn about multiplying a monomial by a trinomial. But first, let’s clarify what monomials and trinomials are. A monomial is an expression that contains only one term, like `3x`. Can anyone provide an example of a trinomial?

How about `4x² + 5x + 7`?

Exactly! Great example, Student_1. Now, when we multiply a monomial with a trinomial, we expand it. This means we multiply the monomial by each term in the trinomial. Remember the acronym 'DPA' for Distribute, Product, and Add. Who can elaborate on how we apply 'DPA'?

We would first distribute the monomial to each term in the trinomial!

Correct! And what do we do next?

Then we find the products of those multiplications!

Exactly! And finally, we add the results together.

Let’s summarize: Multiplying a monomial by a trinomial involves distributing, calculating products, and adding them. Remembering 'DPA' can help us keep track of this process.
Applying the Distributive Law
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson

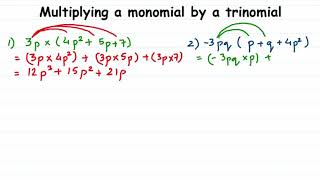
Now that we've reviewed the definitions, let’s put this into practice with an example! Consider `3p × (4p² + 5p + 7)`. What is the first step according to the method we've learned?

We need to distribute `3p` to each term in the trinomial!

Perfect, Student_4! So, what do we get when we multiply?

We get `12p³`, `15p²`, and `21p`.

Excellent job! Now, how do we combine these terms?

We add them together!

Exactly! The result is `12p³ + 15p² + 21p`. Let’s note that this is a polynomial as well. Recap: distribute, multiply, and add!
Visualizing the Process
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson

Let’s draw this out quickly! Imagine the monomial `3p` as a box and the trinomial as another box split into three parts. If we distribute `3p`, what will we visualize?

We would see three different sections, each getting multiplied by `3p`!

That's right! This visual representation helps in understanding the distributive law. Can someone summarize what was drawn?

The `3p` multiplies with each part of the trinomial, showing how each term interacts separately!

Excellent explanation! Visualization helps grasp these concepts more intuitively. Remember: you can always sketch a diagram when you’re stuck!
Consistent Practice
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson

Let’s discuss practice! It’s crucial for mastery. What kinds of problems can we create using our knowledge of multiplying monomials and trinomials?

We could use problems like `x × (2x² + 3x + 4)`!

Or maybe `5y × (y² + 2y + 1)` as practice too!

Fantastic suggestions! Remember, the key is to apply the 'DPA' method each time. Can anyone present a challenge to solve in pairs?

How about `6x × (x² + 2 + 3x)`?

Great choice! Work together and utilize what we've learned. In summary, consistent practice reinforces our skills in multiplying and simplifying these expressions.
Introduction & Overview
Read a summary of the section's main ideas. Choose from Basic, Medium, or Detailed.
Quick Overview
Standard
The section provides a clear explanation of how to multiply a monomial by a trinomial using the distributive law, demonstrating the process through various examples and emphasizing the significance of each term's multiplication and simplification.
Detailed
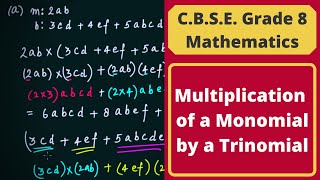
Multiplying a Monomial by a Trinomial
In algebra, multiplication involves not only multiplying numbers but also extending the method to algebraic expressions. A monomial is an expression containing a single term, while a trinomial contains three terms.
To multiply a monomial by a trinomial, we utilize the distributive law, which allows us to multiply each term in the trinomial by the monomial separately. For example, if we take a monomial such as 3p and multiply it by the trinomial 4p² + 5p + 7, we can break this down into:
$$
3p \times (4p² + 5p + 7) = (3p \times 4p²) + (3p \times 5p) + (3p \times 7)
$$
This results in:
12p³from multiplying3pand4p²15p²from multiplying3pand5p21pfrom multiplying3pand7
The final outcome of this multiplication yields a polynomial: 12p³ + 15p² + 21p. This systematic approach not only simplifies the process of multiplying complex expressions but also sets the foundation for polynomial algebra, making further expressions easier to handle. In summary, mastering this technique is vital for solving more advanced algebraic expressions.
Youtube Videos







Audio Book
Dive deep into the subject with an immersive audiobook experience.
Introduction to Multiplying Monomials by Trinomials
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Consider 3p × (4p2 + 5p + 7). As in the earlier case, we use distributive law;
Detailed Explanation
In this section, we are learning how to multiply a monomial by a trinomial. A monomial is a single term like '3p', while a trinomial has three terms like '(4p^2 + 5p + 7)'. To perform this multiplication, we will use the distributive law, which tells us that we need to multiply the monomial by each term in the trinomial individually.
Examples & Analogies
Think of the monomial as the price of one item and the trinomial as a total cost that consists of different components. For example, if the item costs '3p' and the components of the total cost are '4p^2' (for shipping), '5p' (for handling), and '7' (for taxes), we would calculate the total cost by multiplying the price and adding up all the individual costs.
Applying the Distributive Law
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
3p × (4p2 + 5p + 7) =(3p × 4p2) + (3p × 5p) + (3p × 7)
Detailed Explanation
Following the distributive law, we multiply '3p' by each term in the trinomial separately. The first multiplication is 3p × 4p² which gives us 12p³. The second is 3p × 5p which results in 15p². The last multiplication is 3p × 7 resulting in 21p. By performing these calculations step by step, we can simplify our expression.
Examples & Analogies
Picture a worker (3p) who does three different tasks: transporting items (4p²), sorting them (5p), and delivering them (7). The total contribution of the worker for each task shows you how to multiply the worker's unit rate (monomial) by the total tasks (trinomial) he performs.
Combining the Products
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
=12p³ + 15p² + 21p
Detailed Explanation
After performing the individual multiplications, we sum up all the products to get the final result: 12p³ + 15p² + 21p. Each term represents a different part of the overall multiplication, and it's crucial to keep them separate because they cannot be simplified further since they are not like terms.
Examples & Analogies
Imagine you have completed three different projects in a week, and you want to know your total contribution. Each project had a different scale of work. Just like you combine your contributions from these projections (12p³ for large tasks, 15p² for medium tasks, and 21p for small tasks), here we combine all the product terms from our multiplication.
Revisiting the Distributive Law
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Multiply each term of the trinomial by the monomial and add products.
Detailed Explanation
This emphasizes the importance of the distributive law in multiplication, where a single term (monomial) is distributed to each term of the polynomial (trinomial). It helps in achieving clarity in multiplication and ensures no steps are skipped in computation.
Examples & Analogies
Think of distributing snacks among a group of friends. If each friend (a term in the trinomial) receives an equal share of snacks (the monomial), it's essential to ensure every friend gets their fair share rather than skipping anyone. Similarly, by applying the distributive law, we ensure every term in the trinomial receives the monomial's respect smoothly.
Definitions & Key Concepts
Learn essential terms and foundational ideas that form the basis of the topic.
Key Concepts
-
Distributive Law: The technique used to multiply each term in a polynomial by a monomial.
-
Simplifying Expression: The process of combining like terms to present the multiplication result in a simplified format.
-
Term-by-Term Multiplication: The method of multiplying each term of a trinomial by a monomial to obtain the final polynomial.
Examples & Real-Life Applications
See how the concepts apply in real-world scenarios to understand their practical implications.
Examples
-
Multiply
3pby4p² + 5p + 7: Result is12p³ + 15p² + 21p. -
Multiply
xby2x² + 3x + 5: Result is2x³ + 3x² + 5x.
Memory Aids
Use mnemonics, acronyms, or visual cues to help remember key information more easily.
🎵 Rhymes Time
-
When multiplying, take a cue, Distribute first, it's easy to do!
📖 Fascinating Stories
-
Imagine a shop where a single item price is multiplied by several customers’ carts—a clear view of distribution!
🧠 Other Memory Gems
-
'DPA' stands for Distribute, Product, Add—keep multiplying until you’re glad!
🎯 Super Acronyms
DPA = Distribute, then Product, and finally Add.
Flash Cards
Review key concepts with flashcards.
Glossary of Terms
Review the Definitions for terms.
-
Term: Monomial
Definition:
An algebraic expression that contains only one term.
-
Term: Trinomial
Definition:
An algebraic expression that consists of three terms.
-
Term: Distributive Law
Definition:
A property of multiplication over addition or subtraction, allowing the multiplication of a single term by each term in a sum or difference.
-
Term: Polynomial
Definition:
An algebraic expression formed from one or more monomials.

