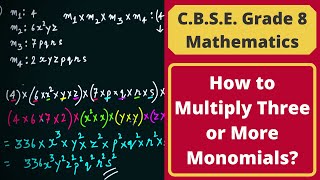
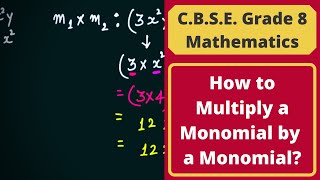
8.3.1 - Multiplying two monomials
Enroll to start learning
You’ve not yet enrolled in this course. Please enroll for free to listen to audio lessons, classroom podcasts and take practice test.
Interactive Audio Lesson
Listen to a student-teacher conversation explaining the topic in a relatable way.
Basics of Monomials
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

Today we will start with monomials. A monomial is a single term that contains a coefficient and one or more variables. Can anyone give me an example of a monomial?

How about `3x`?

Exactly! `3x` is a monomial. What do you think `0xy` is? Is it a monomial?

No, because it has a coefficient of zero, so it equals zero!

Right! Now let's move to multiplying monomials.
Multiplying Coefficients
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

When multiplying two monomials like `3x` and `4y`, what do we do with the coefficients?

We multiply them together!

Correct! So how would we decide what the new coefficient would be for `3x * 4y`?

We would multiply `3` and `4`, giving us `12`.

Great job! Now if we multiply `3x` by `-2y`, what's our result?

It would be `-6xy`!
Combining Variables
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

Now let’s look at the variables. When multiplying, what’s the rule for combining the variables?

We add the exponents of like variables.

Correct! So if we multiply `x^2` and `x^3`, what do we get?

`x^{2+3}` which is `x^5`.

Excellent! Remember the rule: a^m * a^n = a^{m+n}.
Examples in Context
🔒 Unlock Audio Lesson
Sign up and enroll to listen to this audio lesson

Let’s do some examples. What is `5x * 3x^2`?

It’s `15x^{1+2}` which equals `15x^3`.

Fantastic! Now what about `5x * -4xy`?

That’s `-20x^{1+1}y`, which simplifies to `-20x^2y`.

Exactly! Let’s remember these steps as we tackle more complex multiplication.
Introduction & Overview
Read summaries of the section's main ideas at different levels of detail.
Quick Overview
Standard
The section covers the process of multiplying two monomials, explaining the importance of multiplication rules for coefficients and variables. It demonstrates various examples to illustrate how to deal with negative signs and the properties of exponents.
Detailed
Multiplying Two Monomials
In algebra, a monomial is a single term consisting of a coefficient and one or more variables raised to non-negative integer powers. When multiplying two monomials, we use the distributive property and the laws of exponents.
The general rule for multiplication of monomials involves multiplying the coefficients (numerical parts) together and then multiplying the variable parts, adding the exponents of any like variables.
- Basic Examples:
- For instance, multiplying
3xby4x^2results in:
3x * 4x^2 = (3 * 4) (x^1 * x^2) = 12x^{1+2} = 12x^3
- Involving a Negative Coefficient:
- Similarly, if one monomial has a negative coefficient, like
-2y, multiplying by5ygives:
-2y * 5y = (-2 * 5)(y^1 * y^1) = -10y^{1+1} = -10y^2
The section also covers how to handle additional variables and constants within monomials. The multiplication operation applies equally regardless of the complexity, whether it's between positive, negative, or zero coefficients and any number of variables.
Overall, understanding how to multiply monomials is fundamental to more complex algebraic expression manipulations such as polynomials.
Youtube Videos








Audio Book
Dive deep into the subject with an immersive audiobook experience.
Introduction to Multiplying Monomials
Chapter 1 of 4
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
We begin with 4 × x = x + x + x + x = 4x as seen earlier. Notice that all the three products of monomials, 3xy, 15xy, –15xy, are also monomials.
Detailed Explanation
When we multiply a number by a variable, such as multiplying 4 by x, we're effectively adding x together 4 times. This gives us 4x. The example also highlights that products like 3xy and 15xy are classified as monomials because they consist of a number multiplied by variables.
Examples & Analogies
Think of multiplying 4 by x as having 4 bags, each containing x apples. When we say '4 times x', we're counting all the apples, which gives us 4x apples in total.
Basic Examples of Multiplying Monomials
Chapter 2 of 4
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
(i) x × 3y = x × 3 × y = 3 × x × y = 3xy
(ii) 5x × 3y = 5 × x × 3 × y = 5 × 3 × x × y = 15xy
(iii) 5x × (–3y) = 5 × x × (–3) × y = 5 × (–3) × x × y = –15xy
Detailed Explanation
When multiplying two monomials, we multiply their coefficients (the numbers) and then multiply their variables. For instance, for 5x and 3y, we multiply 5 and 3 to get 15, and then combine the variables x and y to give us 15xy. If one of the coefficients is negative, such as in the case of 5x and –3y, the final result becomes negative, resulting in –15xy.
Examples & Analogies
Imagine 5 boxes, each containing strawberries (x), and you want to combine them with each of the 3 baskets of cherries (y). If one box also contains a negative 3 cherries, then when you combine them, you end up with a negative count of these cherries in your final mix!
Multiplying Monomials with Variable Powers
Chapter 3 of 4
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
(iv) 5x × 4x² = (5 × 4) × (x × x²) = 20 × x³ = 20x³
(v) 5x × (–4xyz) = (5 × –4) × (x × xyz) = –20 × (x × x × yz) = –20x²yz
Detailed Explanation
In these examples, we also deal with powers of variables. When multiplying like variables, we add their exponents. For example, in 5x and 4x², since the x has an exponent of 1, we add it to the exponent of 2 from x² to get x³, giving us a final product of 20x³. Similarly, in the second example, we multiply the coefficients and add the powers of x as well.
Examples & Analogies
Consider you have 5 containers each filled with 1 liter of liquid (x) and another container with 4 liters (x²). When you combine them, you can think of the liters of liquid not just being in separate containers, but scaling up – each container fully filled compounds the total volume!
Collecting Variable Powers
Chapter 4 of 4
🔒 Unlock Audio Chapter
Sign up and enroll to access the full audio experience
Chapter Content
Observe how we collect the powers of different variables in the algebraic parts of the two monomials. While doing so, we use the rules of exponents and powers.
Detailed Explanation
When multiplying two monomials, it’s crucial to keep track of how we manipulate the exponents of the variables. The rules of exponents tell us that when we multiply powers with the same base, we add the exponents together. So, if we multiply x¹ with x², we end up with x³.
Examples & Analogies
If you view your variable x as a garden where each plant type has an age represented by the exponent, multiplying the plants together means you’re pairing those ages together, allowing for more growth, which is seen in the higher exponent when you plant different mature plants together.
Key Concepts
-
Monomial: An algebraic expression with one term, such as 5x.
-
Coefficient: The numerical factor in a term of a polynomial, e.g., in 4xy the coefficient is 4.
-
Multiplication of Monomials: When multiplying, multiply coefficients and add the exponents of like variables.
-
Distributive Property: a(b + c) = ab + ac, useful when multiplying two monomials.
Examples & Applications
Example: Multiply 3x * 4x^2 = 12x^3.
Example: Multiply -2y * 5y = -10y^2.
Example: Multiply 5a * -6b = -30ab.
Memory Aids
Interactive tools to help you remember key concepts
Rhymes
Multiply the numbers, combine the facts, add the powers, and see the impacts.
Stories
Once upon a time, there lived two numbers, 2 and 3, who wanted to join hands. When they met, they found they could create 6 together. They also summoned their friends x and y to multiply and dance in harmony producing new terms.
Memory Tools
Remember: M.C.A! Multiply Coefficients, Add exponents.
Acronyms
M.M.E
Monomials Multiply Easily!
Flash Cards
Glossary
- Monomial
An algebraic expression consisting of a single term. Examples include
3x,-4y^2,5xyz.
- Coefficient
The numerical factor in a term of a polynomial or monomial. For example, in
4x, the coefficient is4.
- Exponent
A number indicating how many times to multiply the base. For example, in
x^2, the exponent is2, meaningxis multiplied by itself once.
- Variable
A symbol used to represent a quantity that can change, commonly represented by letters like
x,y, etc.
Reference links
Supplementary resources to enhance your learning experience.
