Professional Courses
Industry-relevant training in Business, Technology, and Design to help professionals and graduates upskill for real-world careers.

Categories
Interactive Games
Fun, engaging games to boost memory, math fluency, typing speed, and English skills—perfect for learners of all ages.

Typing
Memory
Math
English Adventures
Knowledge
Enroll to start learning
You’ve not yet enrolled in this course. Please enroll for free to listen to audio lessons, classroom podcasts and take mock test.
Interactive Audio Lesson
Listen to a student-teacher conversation explaining the topic in a relatable way.
Introduction to Elimination Method
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson

Today, we will discuss the elimination method, a systematic technique to solve pairs of linear equations. Can anyone tell me what a linear equation is?

A linear equation is an equation of the first degree in two variables, like ax + by + c = 0.

Great! Now, the elimination method helps us simplify solving these equations by eliminating one variable. Let's remember this with the acronym 'EAS' - Eliminate, Add, Solve. Can anyone guess the first step?

Adjusting the coefficients to make one variable disappear!

Exactly! We need to make the coefficients equal first before we can eliminate one. Let's move to our first example.
Steps of the Elimination Method
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson

Now, let's discuss the steps systematically. Step one: adjust the coefficients. Consider the equations 9x - 4y = 2000 and 7x - 3y = 2000. How can we make the coefficients of 'y' equal?

We could multiply Equation 1 by 3 and Equation 2 by 4 to get the same coefficient for y!

Right on! Once we have the equations ready, we subtract one from the other, which leads us to eliminate 'y'. Who can explain what we mean by 'subtracting'?

It means we remove one equation from the other to simplify our problem!

Perfect! Finally, solving the remaining equation will help us find the value of our variables. This method is very systematic. Let's practice it with examples after!
Application of the Elimination Method
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson

Let's apply the elimination method to real-world situations. For instance, if we have the incomes and expenditures of two individuals stated in equations, how would we proceed?

We can write the equations based on their incomes and savings!

Then we can eliminate one variable to find out how much each one earns.

Absolutely! And remember to verify your answers by checking the relationships given in the problem.

That makes sense! We can cross-check for consistency after we get our results.

Exactly! Let's dive into some practice problems together now.
Introduction & Overview
Read a summary of the section's main ideas. Choose from Basic, Medium, or Detailed.
Quick Overview
Standard
In this section, we explore the elimination method as an effective alternative to the substitution method for solving pairs of linear equations. By manipulating the equations to eliminate one variable, we can find unique solutions or determine if systems of equations are inconsistent or dependent.
Detailed
Detailed Summary
The elimination method is a powerful algebraic technique used for solving pairs of linear equations. It aims to eliminate one variable by manipulating the equations through addition or subtraction after ensuring equal coefficients for that variable. The standard steps for applying the elimination method include:
- Adjusting Coefficients: Each equation is multiplied by appropriate constants, if necessary, to make the coefficients of a chosen variable (either x or y) equal.
- Eliminating Variables: By adding or subtracting the equations, one variable is eliminated, resulting in a simpler equation in one variable.
- Solving the Remaining Equation: The equation is solved for the remaining variable, which is then substituted back into one of the original equations to find the other variable.
- Identifying Types of Solutions: If a true statement results (like 0 = 0), the system is dependent with infinite solutions. If a false statement appears (like 0 = 9), the system is inconsistent with no solution.
For illustration, we solve real-world problems, such as calculating incomes based on savings, or finding two-digit numbers whose digits differ, applying the method through multiple examples. The significance of the elimination method lies in its ability to simplify complex systems into manageable equations, making it easier to visualize and solve.
Example:
Use the elimination method to find all possible solutions of the following pair of linear equations:
$$
3x + 5y = 11 \quad (1)
5x + 7y = 19 \quad (2)
$$
Solution:
Step 1: Multiply Equation (1) by 5 and Equation (2) by 3 to make the coefficients of \(x\) equal. Then we get the equations as:
$$
15x + 25y = 55 \quad (3)
15x + 21y = 57 \quad (4)
$$
Step 2: Subtracting Equation (4) from Equation (3), we have:
$$
(15x + 25y) - (15x + 21y) = 55 - 57
$$
$$
4y = -2
$$
Thus,
$$
y = -\frac{1}{2}, ext{ which produces unique solutions.}
$$
Therefore, the pair of equations has a unique solution.
Youtube Videos






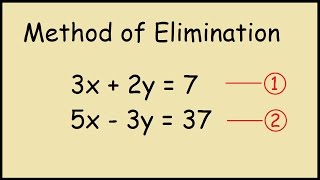









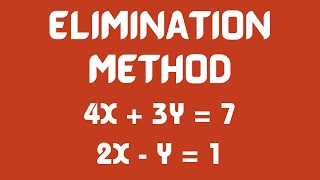



Audio Book
Dive deep into the subject with an immersive audiobook experience.
Introduction to the Elimination Method
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Now let us consider another method of eliminating (i.e., removing) one variable. This is sometimes more convenient than the substitution method. Let us see how this method works.
Detailed Explanation
The Elimination Method focuses on removing one of the variables from a pair of linear equations to simplify the solving process. Instead of substituting one variable into the other equation (as in the substitution method), we manipulate the equations to eliminate one variable entirely.
Examples & Analogies
Imagine you have a complex recipe with multiple ingredients. Sometimes, it's easier to tackle one ingredient at a time. Similarly, the elimination method allows you to deal with one variable first, making it easier to solve the equations.
Example 8 – Solving a Real-World Problem
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Example 8: The ratio of incomes of two persons is 9 : 7 and the ratio of their expenditures is 4 : 3. If each of them manages to save 2000 per month, find their monthly incomes. Solution: Let us denote the incomes of the two person by 9x and 7x and their expenditures by 4y and ` 3y respectively. Then the equations formed in the situation is given by : 9x – 4y = 2000 (1) and 7x – 3y = 2000 (2)
Detailed Explanation
In this example, we first express the incomes and expenditures of the two persons in terms of variables. We then create two equations based on given ratios and their savings. Using elimination, we manipulate these equations to solve for the incomes of the two individuals.
Examples & Analogies
Think of it like solving a mystery with two suspects (the two persons). Each suspect has clues (incomes and expenditures) and by logically eliminating possibilities (variables), you reveal the actual identities (incomes) of the suspects.
Steps of the Elimination Method
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Step 1: Multiply both the equations by some suitable non-zero constants to make the coefficients of one variable (either x or y) numerically equal. Step 2: Then add or subtract one equation from the other so that one variable gets eliminated. If you get an equation in one variable, go to Step 3. If in Step 2, we obtain a true statement involving no variable, then the original pair of equations has infinitely many solutions.
Detailed Explanation
The elimination method consists of systematic steps. First, you ensure the coefficients of one variable are equal; then, you either add or subtract the equations to eliminate that variable. After that, you solve the resulting equation. If the result is true (like 0 = 0), it means the equations have infinite solutions. If it's false (like 0 = 5), they have no solutions.
Examples & Analogies
Imagine you’re cleaning a room (the equations) by removing items (the variables). First, you group similar items (equalize coefficients), then you make your task easier by taking out a full bag of cookies (removing the variable). The end result will tell you whether you can reuse items (infinite solutions) or you've run out of space (no solutions).
Example 9 – No Solution Case
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Example 9: Use elimination method to find all possible solutions of the following pair of linear equations: 2x + 3y = 8 (1) 4x + 6y = 7 (2)
Detailed Explanation
In this example, we set up our equations to equalize the 'x' coefficients by multiplying the first equation. When we subtract the modified equations, we arrive at a false statement, showing that the two lines represented do not intersect and indicating that there is no solution.
Examples & Analogies
Visualize two trains running on parallel tracks. No matter how fast or slow they go, they will never meet (no solution). This situation is like our equations where they represent two lines that never intersect.
Example 10 – Finding Two-Digit Numbers
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Example 10: The sum of a two-digit number and the number obtained by reversing the digits is 66. If the digits of the number differ by 2, find the number.
Detailed Explanation
In this case, we first represent the number in terms of its digits and set up equations based on the given information. By manipulating these equations using the elimination method, we can derive the possible values for the digits and thus identify the two-digit numbers.
Examples & Analogies
Consider finding combinations of your favorite two types of fruit in a basket. Each arrangement (the numbers) must satisfy certain conditions (sum and difference), and by testing different combinations (applying the elimination), you discover which ones work!
Definitions & Key Concepts
Learn essential terms and foundational ideas that form the basis of the topic.
Key Concepts
-
Elimination Method: A technique for solving linear equations by eliminating one variable.
-
Adjusting Coefficients: Multiplying equations to make coefficients of one variable equal.
-
Inconsistent Systems: Systems that have no solutions.
-
Dependent Systems: Systems that have an infinite number of solutions.
Examples & Real-Life Applications
See how the concepts apply in real-world scenarios to understand their practical implications.
Examples
-
Example 1: Solve the equations 9x - 4y = 2000 and 7x - 3y = 2000 to find incomes.
-
Example 2: Use elimination to determine a two-digit number from given conditions.
Memory Aids
Use mnemonics, acronyms, or visual cues to help remember key information more easily.
🎵 Rhymes Time
-
Eliminate, add, then solve with glee, for linear equations, it's the key!
📖 Fascinating Stories
-
Once a wise baker wanted to divide his dough evenly among two loaves. He used a clever method to eliminate extra bits and ensured both breads were perfect!
🧠 Other Memory Gems
-
E.A.S. - Eliminate, Add, Solve to remember the elimination method steps.
🎯 Super Acronyms
EAS - For 'Eliminate, Adjust, Solve' to remember the flow of the elimination method.
Flash Cards
Review key concepts with flashcards.
Glossary of Terms
Review the Definitions for terms.
-
Term: Elimination Method
Definition:
An algebraic technique used to solve pairs of linear equations by eliminating one variable through addition or subtraction.
-
Term: Linear Equation
Definition:
An equation of the first degree in two variables, typically represented in the form ax + by + c = 0.
-
Term: Coefficients
Definition:
Numerical factors in front of variables in an equation.
-
Term: Inconsistent System
Definition:
A system of equations that has no solution due to contradictory conditions.
-
Term: Dependent System
Definition:
A system of equations that has infinitely many solutions because the equations represent the same line.

