Professional Courses
Industry-relevant training in Business, Technology, and Design to help professionals and graduates upskill for real-world careers.
Categories
Interactive Games
Fun, engaging games to boost memory, math fluency, typing speed, and English skillsβperfect for learners of all ages.
Typing
Memory
Math
English Adventures
Knowledge
Enroll to start learning
Youβve not yet enrolled in this course. Please enroll for free to listen to audio lessons, classroom podcasts and take mock test.
Interactive Audio Lesson
Listen to a student-teacher conversation explaining the topic in a relatable way.
Understanding Angles of Elevation
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson
Today, we are going to explore how to find the height of objects using angles of elevation. Can anyone tell me what an angle of elevation is?
Isn't it the angle between the horizontal line and the line of sight when looking up?
Exactly! When you look up at an object, the angle you create with the horizontal line is the angle of elevation. In our example, the observer looks up at the chimney, measuring at 45 degrees. This helps us calculate its height.
Wait! Why do angles help us measure height?
Great question! We can use trigonometric ratios. In this case, we can set up a right triangle to relate the angles and the distances involved!
So, we can find the height just by knowing the angle and the distance?
Yes! And thatβs the power of trigonometry. Let's move on to setting up the right triangle for this scenario.
Setting Up the Triangle
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson
Now, let's set up our triangle. We have the chimney represented as AB and the observer's height as BE. Who remembers the height of the observer?
The observer is 1.5 meters tall!
That's correct! So, AE will be the height from the observer's line of sight to the top of the chimney, which we need to find. Now, what distance do we have?
The observer is 28.5 meters away from the chimney.
Right! So, we can express AB as AE plus the observer's height. We combine these to find the total height of the chimney. Letβs calculate AE using the tangent function. What is the tangent of 45 degrees?
Itβs 1!
Exactly! So we set it up as tan(45Β°) = AE/DE, which helps us calculate the ratios.
Calculating the Height
Unlock Audio Lesson
Signup and Enroll to the course for listening the Audio Lesson
Now we've established that tan(45Β°) = AE/28.5 m. Since we know that tan(45Β°) is 1, we can say AE = 28.5 m. Can anyone remind me how to find the total height of the chimney?
We add AE to the height of the observer!
Exactly! So what is AB β the total height of the chimney?
Itβs 30 meters because AE is 28.5 meters plus the observer's height of 1.5 meters!
Well done! This demonstrates how we can use trigonometry to calculate heights in real-world situations.
Introduction & Overview
Read a summary of the section's main ideas. Choose from Basic, Medium, or Detailed.
Quick Overview
Standard
In this example, an observer measures the angle of elevation to the top of a chimney from a distance. Using trigonometric functions, specifically the tangent of the angle, we determine the height of the chimney based on the observer's height and distance from the chimney.
Detailed
Example 3: Calculating the Height of a Chimney Using Trigonometry
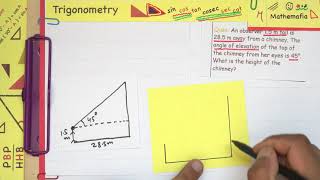
In this example, we explore how to determine the height of a chimney based on an observer's distance from it and the angle of elevation to its top. The observer is 1.5 meters tall and positioned 28.5 meters away from the chimney. The angle of elevation, measured from the observer's line of sight, is 45 degrees.
To solve this problem, we recognize that the scenario can be represented as a right triangle where:
- AB (the chimney's height) can be broken down into two parts: the height above the observer's eyes (AE) and the observer's height (BE).
- The horizontal distance from the observer to the base of the chimney is represented by DE.
Using the tangent ratio, we have the relationship: tan(angle) = opposite/adjacent. Here, tan(45Β°) = AE / DE. Since tan(45Β°) = 1, we find that AE = DE = 28.5 m.
Combining this with the height of the observer, the total height of the chimney (AB) is calculated as: AB = AE + BE = 28.5 m + 1.5 m = 30 m.
Thus, the height of the chimney is 30 meters. This example highlights the practical application of trigonometric ratios in solving real-world measurement problems.
Youtube Videos
Audio Book
Dive deep into the subject with an immersive audiobook experience.
The Scenario
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
An observer 1.5 m tall is 28.5 m away from a chimney. The angle of elevation of the top of the chimney from her eyes is 45Β°.
Detailed Explanation
In this scenario, we have an observer who is standing away from a chimney. The observer's height is 1.5 meters, and she is located 28.5 meters away from the base of the chimney. The angle created between her line of sight to the top of the chimney and the horizontal line from her eyes to the base of the chimney is called the angle of elevation. Here, this angle is 45 degrees.
Examples & Analogies
Imagine you are standing in a park looking up at a tall slide. The distance you are standing from the slide is like the distance to the chimney, and the height of the slide represents the height of the chimney. If you look up at the top of the slide at a 45-degree angle, thatβs similar to what the observer is experiencing.
Understanding the Geometry
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Here, AB is the chimney, CD the observer, and β ADE the angle of elevation. In this case, ADE is a triangle, right-angled at E and we are required to find the height of the chimney.
Detailed Explanation
We can visualize this scenario using a right triangle. In this case, AB represents the height of the chimney, and CD is the observer's line at eye level. The angle of elevation β ADE indicates the angle formed by the horizontal line of the observer's sight and the line extending from her eyes up to the top of the chimney. Since we know one angle (45 degrees) and the two sides opposite and adjacent to it (the observer's distance from the chimney and the height we need to find), we can use trigonometry to solve for AB.
Examples & Analogies
Think of the scenario as trying to find how high a tree is from a specific distance. If you stand at a certain spot and stare upwards at the top of the tree, you create a triangle with the ground and your line of sight. Just like in our triangle situation, we can use objects readily available in the park to visualize angles and heights.
Using Trigonometric Ratios
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
We have AB = AE + BE = AE + 1.5 and DE = CB = 28.5 m. To determine AE, we choose a trigonometric ratio, which involves both AE and DE. Let us choose the tangent of the angle of elevation.
Detailed Explanation
In this part, we express the height of the chimney (AB) in terms of AE, which is the height from the observer's eye level to the top of the chimney. We note that BE is the observer's height (1.5 m), so AB equals AE plus 1.5 m. We then focus on triangle ADE and use the tangent of the angle of elevation to relate AE and DE. The tangent of an angle in a right triangle gives us the ratio of the opposite side to the adjacent side. Since the angle is 45 degrees, we use the tangent function: tan(45Β°) = AE/DE.
Examples & Analogies
If you think back to the tree example, if you wanted to know how high the tree is without climbing it, you could measure a distance from the base and then calculate the height using the angle you see it from. In this case, the height corresponded with the vertical rise (height of the tree) and the horizontal distance you walked away from it.
Calculating the Height
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
Now, tan 45Β° = AE/DE, i.e., 1 = AE/28.5. Therefore, AE = 28.5.
Detailed Explanation
Here, we calculate AE more explicitly using the fact that the tangent of 45 degrees equals 1. This means that the height AE is equal to the distance DE (28.5 m) because the tangent ratio gives us a simplistically direct relationship in this case. Thus, we conclude that AE is 28.5 m.
Examples & Analogies
Going back to our tree analogy, finding AE is like realizing that for every meter you stand from the tree to see it, the height goes up by that same ratio because the angle creates an even slope.
Final Height of the Chimney
Unlock Audio Book
Signup and Enroll to the course for listening the Audio Book
So the height of the chimney (AB) = (28.5 + 1.5) m = 30 m.
Detailed Explanation
Finally, we calculate the total height of the chimney (AB) by adding the height from the observer's eye level (AE) to the observer's height (1.5 m). Therefore, AB equals 28.5 meters plus 1.5 meters, resulting in a total chimney height of 30 meters.
Examples & Analogies
This can be compared to measuring the total height of a tree after determining how high one could see from a point below. You measure from where your eyes are level and add your height to grasp the total height of the tree, which is how we concluded the overall height of the chimney.
Definitions & Key Concepts
Learn essential terms and foundational ideas that form the basis of the topic.
Key Concepts
-
Height Calculation: The process of determining the height of an object using the angle of elevation and distance.
-
Trigonometric Ratios: Functions like tangent that relate angle measures to side lengths in right triangles.
-
Right Triangle Properties: Understanding the relationship between the sides and angles in right triangles.
Examples & Real-Life Applications
See how the concepts apply in real-world scenarios to understand their practical implications.
Examples
-
An observer standing 1.2 meters tall wants to find the height of a tree 35 meters away with an angle of elevation of 60 degrees. Using the tangent function, we can determine the height.
-
If a building is observed from a distance of 50 meters with an angle of elevation of 30 degrees, what would be its height using trigonometric ratios?
Memory Aids
Use mnemonics, acronyms, or visual cues to help remember key information more easily.
π΅ Rhymes Time
-
To find the height of a tower or tree, just look up high and let angles be free.
π Fascinating Stories
-
Once there was a curious observer who hailed from a hill. She measured a chimney tall and got quite a thrill. With angles and distance and her height in the way, she calculated the total height without any delay.
π§ Other Memory Gems
-
A mnemonic to remember: AE is added to BE for total height, A + B = all right.
π― Super Acronyms
Use H = A + B for height calculation
- H: for height
- A: for above eyes
- B: for below.
Flash Cards
Review key concepts with flashcards.
Glossary of Terms
Review the Definitions for terms.
-
Term: Angle of Elevation
Definition:
The angle between the horizontal and the line of sight when looking up at an object.
-
Term: Tangent
Definition:
A trigonometric ratio used to relate the angle of elevation to the opposite side and adjacent side of a right triangle.
-
Term: Right Triangle
Definition:
A triangle that has one angle measuring 90 degrees.
-
Term: Observer
Definition:
A person or entity viewing an object from a specified distance and height.