Pythagorean Triplets

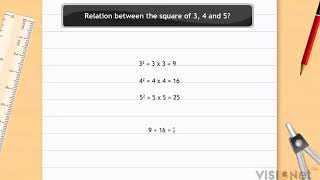
A Pythagorean triplet consists of three positive integers a, b, and c, such that the relationship a² + b² = c² holds true. The simplest example is the triplet (3, 4, 5), where 3² + 4² = 9 + 16 = 25 = 5². Another known triplet is (6, 8, 10) following the same property as 6² + 8² = 36 + 64 = 100 = 10².

Further, the section encourages students to find additional triplets and presents a formula for generating Pythagorean triplets for any natural number m greater than 1:

Form: (2m, m² - 1, m² + 1)

Examples are provided to elaborate on how to derive triplets using this formula, like transforming the number 8 into the triplet (8, 15, 17). The significance of Pythagorean triplets extends beyond mathematics and is useful in various applications, such as architecture and physics, to determine lengths and distances.

Similar Questions

1. Write a Pythagorean triplet whose smallest member is 5.

Solution: We can get Pythagorean triplets by using the general form $2m, m^2 - 1, m^2 + 1$.
Let us first take
$$m^2 - 1 = 5$$
So,
$$m^2 = 5 + 1 = 6$$
which gives
$$m = \sqrt{6} \text{ (not an integer)}$$
Therefore, let us try
$$2m = 2$$
$$m = 1$$
Then we get
$$2m = 2 \quad \text{and} \quad 1^2 - 1 = 0 \quad \text{and} \quad 1^2 + 1 = 2$$
The triplet is 2, 0, 2 with 0 as the smallest member, so let us try something else.

1. Write a Pythagorean triplet whose smallest member is 12.

Solution: We can use the general form $2m, m^2 - 1, m^2 + 1$.
Let’s first take
$$m^2 - 1 = 12$$
So,
$$m^2 = 13$$
which gives
$$m = \sqrt{13} \text{ (not an integer)}$$
Therefore, let us try
$$2m = 6$$
$$m = 3$$
Then we get
$$2m = 6 \quad \text{and} \quad 3^2 - 1 = 8 \quad \text{and} \quad 3^2 + 1 = 10$$
The triplet is 6, 8, 10 with 6 being the smallest member.

1. Write a Pythagorean triplet where the smallest member is 15.

Solution: We can derive Pythagorean triplets using the general form $2m, m^2 - 1, m^2 + 1$.
Let’s first evaluate
$$m^2 - 1 = 15$$
So,
$$m^2 = 16$$
which gives
$$m = 4$$
Thus, we have
$$2m = 8 \quad \text{and} \quad 4^2 - 1 = 15 \quad \text{and} \quad 4^2 + 1 = 17$$
Hence, the triplet is 8, 15, 17 with 8 being the smallest member.

1. Write a Pythagorean triplet whose smallest member is 7.

Solution: We can obtain Pythagorean triplets using the format $2m, m^2 - 1, m^2 + 1$.
Let us initially set
$$m^2 - 1 = 7$$
So,
$$m^2 = 8$$
which gives
$$m = \sqrt{8} \text{ (not an integer)}$$
Thus, let us examine
$$2m = 4$$
$$m = 2$$
Then we compute
$$2m = 4 \quad \text{and} \quad 2^2 - 1 = 3 \quad \text{and} \quad 2^2 + 1 = 5$$
The triplet is 4, 3, 5 with 3 being the smallest member.